3D printed scaled setup for smoke transport analysis in a subterranean passenger platform

Theoretical smoke transport simulation in a subterranean passenger platform using CFD

R. Rivera-Blas, L. A. Flores-Herrera, J. Humberto Pérez-Cruz, N. Muñoz-Aguirre, C. Torres-Martínez, M. F. Carbajal-Romero, R. Vargas-Carmona, M. Funes-Lora

Instituto Politécnico Nacional, Sección de Estudios de Posgrado e Investigación, Escuela Superior de Ingeniería Mecánica y Eléctrica, Unidad Azcapotzalco: Av. de las Granjas No. 682 Col. Santa Catarina, c. p. 02250, Ciudad de México, México.

rriverab@ipn.mx, lafloresh@ipn.mx, jhperez@ipn.mx, nmunoz@ipn.mx, ctorresm@ipn.mx, mcarbajalr@ipn.mx, rvargasc1400@egresado.ipn.mx, miguelaf@umich.edu

Corresponding author: lafloresh@ipn.mx

Abstract

The mechanism of smoke transport in a subterranean installation is investigated here by means of a CFD simulation. The results show the displacement pattern of the smoke in free motion and compared with the influence of an induced ventilation velocity (critical velocity). The comparison of results was carried using ANSYS-Fluent and FDS computer programs. Individual CAD models were constructed for each case considering real dimensions of a passenger platform. The simulation considers the emission of smoke produced by a totally developed 5MW fire source during 180 seconds. The critical velocity was calculated considering NFPA 130-7. The evaluation of the critical velocity was first validated with a theoretical model. The direction of the smoke inside the platform was observed with respect to time for both cases. The connections of the passenger platform environment with the atmospheric pressure are one of the most important elements to consider in ventilation systems, especially when emergency procedures of complex scenarios have to be designed. These results can be used in the development and location of safety zones for the passengers, to reduce the risk of toxic gases inhalation and to identify possible escape routes in the case of fire.

Keywords: CFD, FDS, ANSYS-FLUENT, Kennedy model, Smoke, Fire, Critical speed.

1. Introduction

Comparison of works related to simulation of smoke in subway platform with the use of FLUENT and FDS have been widely reported [1]. Fire disasters around the world have caused the emergence of numerous studies to analyze response procedures in subway fire emergencies [2]. This includes the interaction of all elements involved in the site [3], before and after the emergency is presented. Fire safety is a matter that should be left not only to the local security personnel or firefighters because of the complexity of the situations, for example in the case of evacuation procedures [4, 5].

Calculation and simulation tools like Computational Fluid Dynamics (CFD) have provided great contributions for the discrete representation of this kind of physical scenarios [6, 7]. Fires presented in subterranean tunnels and underground facilities can be analyzed using different theoretical approaches. These kind of disasters involve a great human cost because in subterranean emergency sceneries, the possibilities for the passengers to leave or scape out of a critical location is quite compromised.

The limited space for mobility, low visibility, presence of fresh air, etc., are some of the restrictions that a passenger can face in this situation. The study presented here considers the presence of smoke inside of a subway passenger platform located in Mexico City with a particular set of ventilation ports connected to atmospheric pressure. Their original dimensions and main architectural details were considered for modeling the analysis scenario.

Connections to atmospheric pressure in subterranean installations are one of the most important issues that have to be considered when designing ventilation systems. The location and size of the ventilation ports, weather conditions, and external air velocity among others create set of variable air conditions. In the case of subway tunnels, convective flow currents enhance the generation of vortex in random locations even when the air velocity can be maintained constant along the tunnel by natural conditions or by means of a mechanical ventilation system. The emission of smoke close to a ventilation duct connected to the atmosphere does not always guarantee a safety condition. The atmospheric pressure creates an equilibrium between the internal and external pressures and the resulting direction of the air flow in consequence is not easy to predict by a direct observation.

2. Previous considerations

The location of the smoke source considered for this analysis was selected close to the center of the platform [7], the fire source is considered as totally developed from the beginning of the simulation and some details on the architecture modeling were simplified as in the case of the access stairs which were substituted by inclined planes. The evacuation of the smoke trough the natural ventilation ports is another interesting issue to observe in our simulation [8] and to notice the presence or not of the backlayering effect with the considered critical velocity [9-11]. The results obtained in [12] compare mathematical models and the possibility of using FLUENT and FDS to establish the parameters that could be used in a smoke transportation study.

3. Methodology

The study was analyzed considering five cases. On the first case, the incompressible Navier-Stokes equations were implemented in FORTRAN to observe the idealized behavior of the analytical model. On the second and third cases, 3D models were created for ANSYS-FLUENT and for the Fire Dynamic Simulator (FDS) software to analyze the air flow behavior.

The fourth case includes a 5 MW fire source moving under free fire conditions. In the fifth case the interaction of the air flow in the smoke behavior was observed during 180 seconds [13]. In all models, the geometric details and dimensions of the platform were considered according real dimensions. The first two cases were analyzed in stationary state and the last three were simulated with respect to time. The critical velocity is calculated with NFPA 130-7, and the obtained value is used for the analytical and numerical models (except for case 4), the analyzed cases are listed in table I.

Table I. List of the analyzed cases.

Case No.	Program	Simulation type	Objective
1	Fortran	Air flow at critical velocity	Validation
2	ANSYS-Fluent	Air flow at critical velocity
3	FDS	Air flow at critical velocity
4	FDS	Smoke at free motion condition	Differentiate the influence of the air flow at critical velocity
5	FDS	Smoke transported with the air flow at critical velocity

3.1. Description of the analysis scenario

In the presence of fire inside underground facilities, different parameters should be considered to handle the emergency situation and provide a suitable breading atmosphere for the users, it must be kept sustainable conditions for life. However, every subway system has its own particular risks, it requires specific solutions which relate internal and external conditions of the tunnel as an enclosed place with limited escape routes.

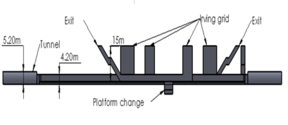
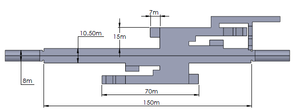
The analyzed platform has 15 m depth of from the sidewalk level and 150 m in length, it has four ventilation ports at the ceiling, connected to atmospheric pressure and three passenger access towards the street. Figure 1, shows the dimensions and geometry considered for the 3D model.

a)	b)

Figure 1. 3D CAD geometry and dimensions a) lateral view and b) top view.

3.2. Critical velocity

During the backlayering effect, the ventilation conditions are not suitable to transport the smoke to a desired direction or exit. The smoke produced by a fire source can be moved then to the opposite direction. The critical velocity has become in one of the prime criteria for the design of ventilation systems, especially in tunnel section [14, 15]. In this study, the Kennedy model (equations 1 up to 3) were used to calculate the critical velocity in the subterranean scenario [16-18]. The value of the critical speed depends on the power of fire, tunnel slope and tunnel cross section; thus, it is determined with the simultaneous solution of the Kennedy model [19].

${V}_{c}={K}_{1}{K}_{2}{\left.{\frac {gHQ}{{P}_{\infty }{C}_{p}{AT}_{F}}}\right.}^{\frac {1}{3}}$
${T}_{F}=\left.{\frac {Q}{{P}_{\infty }{C}_{p}{AV}_{c}}}\right.+{T}_{\infty }$
${K}_{1}={F}_{r}^{-1/3}$

For the model, K₁= 0.605 is a dimensionless constant value given by equation 3, where the Froude number considered for the calculation takes a value of 4.5 according to [20] and K₂=1 is a dimensionless slope factor, the average burning temperature was T_F=369.34 °K (96.2°C), which resulting values correspond to the ones presented in [21]. The 5 MW fire source was considered according with [22, 23]. The remaining values considered for the critical speed calculation are shown in table II.

Table II. Values considered for the critical velocity calculation using the Kennedy model.

Variables	Value
Gravity acceleration (g)	9.81 ${\textstyle {\frac {m}{{s}^{2}}}}$
Tunnel height (H)	5.2 m
Slope	0 %
Air density ( ${\textstyle {P}_{\infty }}$)	1.176 ${\textstyle {\frac {Kg}{{m}^{3}}}}$
Specific heat of air (Cp)	1.007 ${\textstyle {\frac {KJ}{Kg\,{\mbox{°}}K}}}$
Transverse section area (A)	41.6 ${\textstyle {m}^{2}}$
Air temperature ( ${\textstyle {T}_{\infty }}$)	300.15 °K (97 °C)

4. General formulation

4.1. Theoretical analysis implemented in FORTRAN

The present analysis is based on the pressure-velocity coupling method known as vorticity - stream function. The governing equations for the analysis consider an incompressible and Newtonian flow (2D), the conservation of mass (equation 4) and momentum (equation 5) [24]. The analysis contemplates a procedure step by step with respect to time, variable velocities ( ${\textstyle {u}_{i}}$), density ( ${\textstyle \rho }$ ) and dynamic viscosity (μ):

$\left.\nabla \,.\,\,{u}_{i}\right.=0$
${\frac {\partial }{\partial t}}\left.{\rho u}_{i}\right.+{\rho u}_{i}\left.{u}_{i}\cdot \nabla \right.=$$-\nabla p+\mu \,({{\nabla }^{2}u}_{i})$

The vorticity - stream function ( ${\textstyle \omega ,\,\varphi }$ ) equations were discretized using the finite differences method where each node is used to describe the computer dominion (equations 6 and 7) [24]:

${\frac {{\partial }^{2}\varphi }{\partial {x}^{2}}}+\,{\frac {{\partial }^{2}\varphi }{\partial {y}^{2}}}\,=$$-\omega$
${\frac {\partial \omega }{\partial t}}+\,U{\frac {\partial \omega }{\partial x}}\,+$$\,V{\frac {\partial \omega }{\partial y}}=\,{\frac {1}{Re}}\,\left[{\frac {{\partial }^{2}\omega }{\partial {x}^{2}}}+\right.$$\left.\,{\frac {{\partial }^{2}\omega }{\partial {y}^{2}}}\right]$

Here, U and V correspond to the velocities in the x and y direction (eq. 8):

${\textstyle U={\frac {\partial \varphi }{\partial y}}}$ , ${\textstyle V=}$$-{\frac {\partial \varphi }{\partial x}}$

After discretization of equation 6, the following is obtained:

$ax\,{\Psi }_{\left.i-1,\,j\right.}+b\,{\Psi }_{\left.i,\,j\right.}+cx\,{\Psi }_{\left.i+1,\,j\right.}=$$-ay\,{\Psi }_{\left.i,\,\,j+1\right.}-cy\,{\Psi }_{\left.i,\,j-1\right.}-$${\omega }_{\left.i,\,j\right.}$

$ay\,{\Psi }_{\left.i,\,j-1\right.}+b\,{\Psi }_{\left.i,\,j\right.}+cy\,{\Psi }_{\left.i,\,j+1\right.}=$$-ax\,{\Psi }_{\left.i+1,\,\,j\right.}-cx\,{\Psi }_{\left.i-1,\,j\right.}-$${\omega }_{\left.i,\,j\right.}$

In which, the coefficients are:

${\textstyle ax={\frac {1}{\Delta {x}^{2}}}\,,\,\,ay=}$${\frac {1}{\Delta {y}^{2}}}\,,\,\,b=-{\frac {2}{\Delta {x}^{2}}}-{\frac {2}{\Delta {y}^{2}}}\,,\,\,cx=$${\frac {1}{\Delta {x}^{2}}}\,,\,\,cy={\frac {1}{\Delta {y}^{2}}}$

And for equation 7:

$ax\,{\omega }_{\left.i-1,\,j\right.}+b\,{\omega }_{\left.i,\,j\right.}+cx\,{\omega }_{\left.i+1,\,j\right.}=$$-ay\,{\omega }_{\left.i,\,j-1\right.}-cy\,{\omega }_{\left.i,\,j+1\right.}$

$ay\,{\omega }_{\left.i,\,j-1\right.}+b\,{\omega }_{\left.i,\,j\right.}+cy\,{\omega }_{\left.i,\,j+1\right.}=$$-ax\,{\omega }_{\left.i-1,\,j\right.}-cx\,{\omega }_{\left.i+1,\,j\right.}$

The coefficients are:

${a}_{x}=-{\frac {1}{{2\Delta }_{x}}}{\frac {\partial \Psi }{\partial y}}-{\frac {1}{Re{\Delta x}^{2}}}\,,\,{a}_{y}=$${\frac {1}{{2\Delta }_{y}}}{\frac {\partial \Psi }{\partial x}}-{\frac {1}{Re{\Delta y}^{2}}},\,{\,c}_{x}=$${\frac {1}{{2\Delta }_{x}}}{\frac {\partial \Psi }{\partial y}}-{\frac {1}{Re{\Delta x}^{2}}}$ ${c}_{y}=-{\frac {1}{{2\Delta }_{y}}}{\frac {\partial \Psi }{\partial x}}-{\frac {1}{Re{\Delta y}^{2}}},\,b=$${\frac {1}{\Delta t}}+{\frac {2}{Re\Delta {x}^{2}}}+{\frac {2}{Re\Delta {y}^{2}}}$

The resulting Reynolds number is in this case 4.898 x 10^-5.

4.1.1. Boundary conditions in the theoretical model

Figure 2, shows the distribution of boundaries in the 2D model (case 1). The figure represents an idealized duct; the control area, represents a horizontal layer crossing along the center of the passenger platform. The corresponding mesh was formed by 50x275 rectangular divisions. The entire rectangle corresponds to the first 59 m measured from the left side of the platform. The location of each boundary condition is applied on each side by considering the Thom formulation and an idealized velocity profile [25].

Figure 2. Boundary conditions applied to the FORTRAN model

4.1.2. Boundary conditions in ANSYS-FLUENT

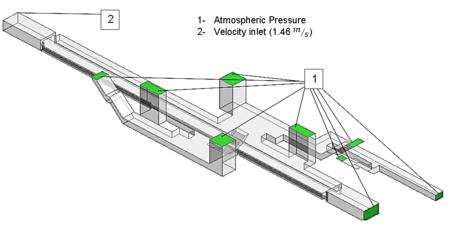
This analysis (case 2) was carried to observe the flow stream along the platform at the critical velocity and compare with case 1. It considers the Finite Volume formulation allowing the discretization and solution of the differential equations. The resulting 3D model is shown in figure 3. The model is composed by a large rectangular shape representing the passenger platform, four rectangular blocks were used to represent the natural ventilation ports. Another three slender blocks (tilted) were used to represent the stairs.

Figure 3. Boundary conditions applied to the 3D ANSYS-FLUENT model.

Different mesh parameters were tested until an excellent mesh quality was obtained according with [26], the resulting model contains 284,816 nodes and 247,974 elements. The location of the areas connected to atmospheric pressure were identified as condition type 1. These includes the natural ventilation ports and passenger accesses. For the type 2 condition, 1.46 m/s of air velocity was applied at one end of the tunnel (left side).

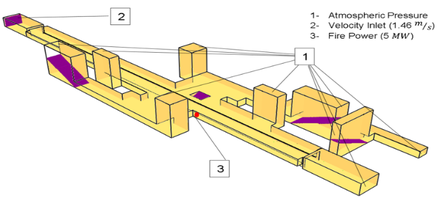
4.1.3. Boundary conditions in FDS

Fire Dynamic Simulator (FDS) was used to simulate the behavior of the calculated critical velocity in the platform [27]. The CAD modelling for this case was created considering a series of coordinates along the X, Y and Z axes as shown in figure 4. The total number of rectangular cells conforming the FDS mesh was 2,474,693. In this model, three case studies were considered, one was used to verify the development of the critical velocity named case 3. The fourth case includes the fire source in free motion and the fifth one includes the fire source and the application of the critical velocity (location 2).

Figure 4. Boundary conditions applied to the 3D FDS model

5. Results

The gathering of results was made considering a horizontally oriented rectangular plane crossing along the model, located at 3.4 m (height) from the floor level, in the middle of the platform, this plane was used to observe all sceneries, now for each case:

5.1. Case 1- Results obtained in FORTRAN

Figure 5 a), shows a dimensionless scale of the resulting air velocity distribution. This results were scaled to the real dimensions and tracked through a crossing line at the middle of the plane as shown in figure 5 b).

a)	b)

Figure 5. Velocity distribution along the idealized duct in FORTRAN.

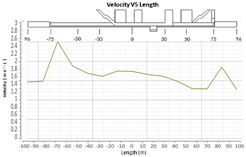
5.1. Case 2- Results obtained in ANSYS-FLUENT

Figure 6 a), shows the resulting velocity contour obtained in ANSYS-FLUENT. The red frame represents the portion of the model analyzed in FORTRAN. An increment of the velocity is present at both ending sides of the model due the reduction of the cross section area (-70m and 80m), this is the connection between the tunnel and the passenger platform.

a)	b)

Figure 6. Contour of velocity distribution (ANSYS-FLUENT).

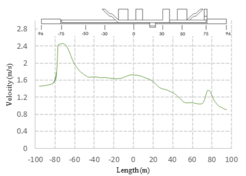
5.2. Case 3. Results obtained in FDS

Figure 7 a), shows the velocity contour obtained in FDS for case 3. The results present a similar behavior as in ANSYS-FLUENT.

a)	b)

Figure 7. Contour of velocity distribution (FDS).

5.4. Comparison between cases 4 and 5

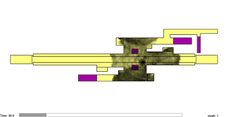
Case 4 corresponds to the simulation of the smoke evolution in free motion condition. The smoke moves only according to the influence of the temperature. Case 5 presents the evolution of the smoke affected with an air velocity of 1.46 m/s. The evolution of the simulations is presented in figure 8. From a) to c) corresponds to case 4 and figures d) to f) corresponds to case 5.

Evolution of smoke for Case 4	Evolution of smoke for Case 5
a) 30 sec.	d) 30 sec.
b) 90 sec.	e) 90 sec.
c) 180 sec.	f) 180 sec.
Figure 7. Evolution of smoke transportation for cases 4 and 5.

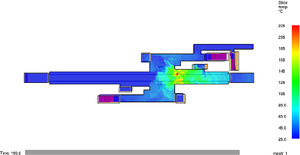
The difference of heat distribution for cases 4 and 5 after 3 minutes of fire evolution is presented in figure 9.

Temperature distribution for case 4	Temperature distribution for case 5
a)	c)
b) 180 sec.	d) 180 sec.
Figure 9. Temperature distribution for cases 4 and 5.

6. Discussion

In the theoretical model, a maximum air velocity value of 2.5 m/s was obtained (cases 1 to 3). This value is similar to the one obtained in ANSYS-FLUENT and in FDS. In case 4, a radial expansion of the smoke (with respect to the fire source) was observed, the movement is reduced when the smoke achieves the natural ventilation ports in which the smoke flows outside the platform. The influence of the critical velocity was observed in case 5. The air stream increases the possibility of having smoke-free areas were the passengers can have better breathing conditions.

7. Conclusions

According to the simulation, 1.46 m/s is required to produce adequate smoke transportation of the 5MW fire source; no backlash was observed. Comparison between cases 4 and 5 show this difference, the flow stream allow to maintain better conditions for the passengers during their possible evacuation trajectory. This was an idealized case in which the velocity of air can be altered by the presence of the piston effect produced by trains traveling along the tunnels. In real case, an increment of the velocity caused by external factors could produce an increment in the combustion due oxygen enrichment to the fire source. The results presented here correspond qualitatively to the ones obtained in [21] and [28] with differences related to the power of fire considered in each investigation. The time it takes the smoke to occupy the entire platform can be used to calculate the available time the passengers have to evacuate the platform as it was presented in [12, 14] in which the critical velocity plays an important role.

8. Acknowledgements

Authors acknowledge the National Polytechnic Institute (IPN) and the National Council of Science and Technology (CONACYT) for the support provided to this academic research.

9. References

[1] Wang Binbin, Comparative Research on FLUENT and FDS’s Numerical Simulation of Smoke Spread in Subway Platform Fire, Procedia Engineering 26 (2011) 1065 – 1075, doi:10.1016/j.proeng.2011.11.2275

[2] Qiming Li, Yongliang Deng, Cong Liu, Qingtian Zeng, Ying Lu, Modeling and analysis of subway fire emergency response: An empirical study, Safety Science 84 (2016) 171–180, http://dx.doi.org/10.1016/j.ssci.2015.12.003 0925-7535

[3] Ran Gao, Angui Li, Ying Zhang, Na Luo, How domes improve fire safety in subway stations, Safety Science 80 (2015) 94–104, http://dx.doi.org/10.1016/j.ssci.2015.07.015 0925-7535

[4] Wang Zhilei, Hua Min, Xu Dayong, Pan Xuhai, Simulation research on human evacuation in subway with a single point fire scenario, Procedia Engineering 84 ( 2014 ) 595 – 602, doi: 10.1016/j.proeng.2014.10.472

[5] Jing-hong Wang, Wen-yu Yan, You-ran Zhi, Jun-cheng Jiang, Investigation of the panic psychology and behaviors of evacuation crowds in subway emergencies, Procedia Engineering 135 ( 2016 ) 128 – 137, doi: 10.1016/j.proeng.2016.01.091

[6] Na Luo, Angui Li, Ran Gao, Zhenguo Tian, Zhipei Hu, Smoke confinement utilizing the USME ventilation mode for subway station fire, Safety Science 70 (2014) 202–210, http://dx.doi.org/10.1016/j.ssci.2014.06.002 0925-7535

[7] Jae Seong Roh, Hong Sun Ryou, Won Hee Park, Yong Jun Jang, CFD simulation and assessment of life safety in a subway train fire, Tunnelling and Underground Space Technology 24 (2009) 447–453, doi:10.1016/j.tust.2008.12.003

[8] Dong-Ho Rie, Myung-Whan Hwang, Seong-Jung Kim, Sung-Wook Yoon, Jae-Woong Ko, Ha-Yong Kim, A study of optimal vent mode for the smoke control of subway station fire, Tunnelling and Underground Space Technology 21 (2006) 300–301, doi:10.1016/j.tust.2005.12.157

[9] Shaogang Zhang, Yongzheng Yao, Kai Zhu, Kaiyuan Li, Ruifang Zhang, Song Lu, Xudong Cheng, Prediction of smoke back-layering length under different longitudinal ventilations in the subway tunnel with metro train, Tunnelling and underground space technology 53 (2016) 13-21, http://dx.doi.org/10.1016/j.tust.2015.12.013 0886-7798.

[10] Shaogang Zhang, Xudong Cheng, Yongzheng Yao, Kai Zhu, Kaiyuan Li, Song Lu, Ruifang Zhang, Heping Zhang, An experimental investigation on blockage effect of metro train on the smoke back-layering in subway tunnel fires, Applied Thermal Engineering 99 (2016) 214–223, http://dx.doi.org/10.1016/j.applthermaleng.2015.12.085 1359-4311

[11] Miao-cheng Weng, Xin-ling Lu, Fang Liu, Xiang-peng Shi, Long-xing Yu, Prediction of backlayering length and critical velocity in metro tunnel fires, Tunnelling and Underground Space Technology 47 (2015) 64–72, http://dx.doi.org/10.1016/j.tust.2014.12.010 0886-7798

[12] Kai Kang, A smoke model and its application for smoke management in an underground mass transit station, Fire Safety Journal 42 (2007) 218–231, doi:10.1016/j.firesaf.2006.10.003

[13] NFPA 130, Standard for Fixed Guideway Transit & Passenger Rail Systems, (2007 edition)

[14] Manabu Tsukahara, Yusuke Koshiba, Hideo Ohtani, Effectiveness of downward evacuation in a large-scale subway fire using Fire Dynamics Simulator, Tunnelling and Underground Space Technology 26 (2011) 573–581, doi:10.1016/j.tust.2011.02.002

[15] Ahmed Kashef, Zhongyuan Yuan, Bo Lei, Ceiling temperature distribution and smoke diffusion in tunnel fires with natural ventilation, Fire Safety Journal 62 (2013) 249–255, http://dx.doi.org/10.1016/j.firesaf.2013.09.019

[16] W. K. Chow, On smoke control for tunnels by longitudinal ventilation, Fire and life safety, Tunnelling and Underground Space Technology, vol. 13, issue 3, (1998), 271–275, http://dx.doi.org/10.1016/S0886-7798(98) 00061-3

[17] Y. Wu, M.Z.A. Bakar, Control of smoke in tunnel fires using longitudinal ventilation systems - a study of the critical velocity, Fire Safety Journal 35 (2000) 363-390, http://dx.doi.org/10.1016/S0379-7112(00)00031-X

[18] Falin Chen, Smoke propagation in road tunnels, Applied Mechanics Reviews, 53 (8), 207-218 (2000), doi:10.1115/1.3097350

[19] W.D. Kennedy, J.A. Gonzalez, J.G. Sanchez, Derivation and application of the SES critical velocity equations, Transactions-American Society of Heating Refrigerating and Air Conditioning Engineers (ASHRAE) vol. 102, pt. 2, (1996), USA ISSN 0001-2505

[20] J. H. Klote, J. A. Milke, P. G. Turnbull, A. Kashef, M. J. Ferreira, Handbook of Smoke Control Engineering. Atlanta. ASHRAE, ICC, SFPE & NFPA, (2012) USA, ISBN 978-1-936504-24-4

[21] E. K. Stefopoulos, D. G. Damigos, Design of emergency ventilation system for an underground storage facility, Tunnelling and Underground Space Technology, 22 (3), 293–302, (2007), http://dx.doi.org/10.1016/j.tust.2006.07.002

[22] Morgan J. Hurley, SFPE Handbook of Fire Protection Engineering, 5th edition, Springer (2016) USA, ISBN-13: 978-1493925643

[23] NFPA 502, Standard for Road Tunnels, Bridges, and Other Limited Access Highways, (2011 edition).

[24] Suhas V. Patankar, Numerical Heat Transfer and Fluid Flow. Series in computational methods in mechanics and thermal sciences, McGraw-Hill, (1980) USA, ISBN: 0-07-048740-5

[25] A. Thom, (1933), The flow past circular cylinders at low speeds, Proc. Roy. Soc. London A 141, 651-669.

[26] ANSYS Fluent Meshing User's Guide, ANSYS Inc., Release 15.0 (2013) USA, http://www.ansys.com

[27] Kevin McGrattan, Bryan Klein, Simo Hostikka, Jason Floyd, Fire Dynamics Simulator User’s Guide (Version 5), NIST Special Publication 1019-5, (2007) USA

[28] Deng Jun, Ma Li, Wang Zhen-ping, Xing Zhen, Wang Wei-feng, Simulation Study on Critical Velocity of Longitudinal Ventilation Tunnel Fire, Procedia Engineering 52 ( 2013 ) 67 – 71, doi: 10.1016/j.proeng.2013.02.107