Yangyu mech (talk | contribs) m (Tag: Visual edit) |
|||
| (28 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
| − | + | <!-- | |
<div class="center" style="width: auto; margin-left: auto; margin-right: auto;"> | <div class="center" style="width: auto; margin-left: auto; margin-right: auto;"> | ||
| Line 14: | Line 14: | ||
<div class="center" style="width: auto; margin-left: auto; margin-right: auto;"> | <div class="center" style="width: auto; margin-left: auto; margin-right: auto;"> | ||
| − | * Corresponding author:Yu Yang,E-mail:y[mailto:angyu_mech@qq.com angyu_mech@qq.com]</div> | + | * Corresponding author:Yu Yang,E-mail:y[mailto:angyu_mech@qq.com angyu_mech@qq.com]</div>--> |
| + | ==Abstract== | ||
| − | + | High-temperature bulk superconductors are widely used in various superconducting devices due to their high critical current density, which enables them to trap high magnetic fields. However, the critical current density of bulk superconductors is influenced by magnetic field, temperature and stress, and both increase of stress and temperature can lead to a decrease in the critical current density. And the influence of magnetic field, current, stress are interactional. In this paper, the multi-field coupled analysis is employed to investigate the electromagnetic and mechanical behavior on bulk superconductor during pulsed field magnetization. The simulation results were compared with the present experimental results. The results showed a good agreement between the simulation and experimental results, further validating the reliability of the numerical simulation method. The magnetic field, current, temperature, and stress distribution and variation within the bulk sample during the pulsed magnetization process were calculated and analyzed. Additionally, the influence of the central crack and edge crack in the bulk superconductor was investigated. The results indicate that the region near the crack experiences a variation in current flow direction, resulting in local temperature rise and significant electromagnetic forces. The tip of the crack exhibits stress concentration, leading to a decrease in critical current density. Bulk supercondutor with edge crack case exhibits greater stress concentration compared to those with central crack case. The impact of crack length on maximum stress was analyzed, revealing that as the crack length increases, both the maximum hoop stress and radial stress within the bulk sample rise. | |
| − | ''' | + | '''Keywords''':Bulk superconductor, multi-field coupled analysis, critical current density, finite element method, crack |
| − | =1 Introduction= | + | ==1. Introduction== |
| − | Due to their high critical current density and ability to trapped higher magnetic fields, high-temperature bulk superconductors have found widespread applications in various superconducting devices<span id='cite-_Ref132794187'></span>[[#_Ref132794187| | + | Due to their high critical current density and ability to trapped higher magnetic fields, high-temperature bulk superconductors have found widespread applications in various superconducting devices <span id='cite-_Ref132794187'></span>[[#_Ref132794187|[1,2,3,4]]]. There are three magnetization methods for bulk superconductor: field cooling (FC), zero-field cooling (ZFC), and pulse field magnetization (PFM). Although FC and ZFC can achieve higher trapped fields, they suffer from drawbacks such as long loading times, high energy losses, and the requirement of expensive equipment <span id='cite-_Ref132811978'></span>[[#_Ref132811978|[5,6,7,8,9]]]. In contrast, the advantages of PFM are simplicity, convenience, and cost-effectiveness. It features extremely short loading times and low energy losses, while imposing minimal requirements on the equipment. However, the limitations of PFM are the rapid loading rate leading to significant temperature rises, flux jumps and making it difficult to achieve high trapped fields <span id='cite-_Ref132814132'></span>[[#_Ref132814132|[10]]]. |
| − | Due to the weak mechanical properties of bulk superconductor and the large Lorentz force in magnetization, the mechanical behavior is important in the practical application. The bulk superconductors are sometimes reinforced externally with metals to improve their mechanical performance and prevent fractures<span id='cite-_Ref132897971'></span>[[#_Ref132897971| | + | Due to the weak mechanical properties of bulk superconductor and the large Lorentz force in magnetization, the mechanical behavior is important in the practical application. The bulk superconductors are sometimes reinforced externally with metals to improve their mechanical performance and prevent fractures <span id='cite-_Ref132897971'></span>[[#_Ref132897971|[11,12,13,14]]]. Ainslie and Fujishiro addressed the modeling issues of magnetizing bulk superconductors and provided a finite element method to assess and predict their magnetization characteristics <span id='cite-_Ref132914481'></span>[[#_Ref132914481|[19]]]. In 2018, Wu et al. investigated the stress distribution in high-temperature superconductors under PFM magnetization process and proposed methods to mitigate stress <span id='cite-_Ref132901240'></span>[[#_Ref132901240|[15]]]. Subsequently, they studied the mechanical behavior of non-uniform high-temperature superconductors under PFM magnetization, analyzing the potential for fracture and damage due to non-uniform distributions and their impacts on performance and lifespan <span id='cite-_Ref132901719'></span>[[#_Ref132901719|[16]]]. Xia et al. studied the characteristics of thermomagnetic instability in MgB<sub>2</sub> bulk samples and provided numerical simulation methods to evaluate and predict their performance <span id='cite-_Ref132914048'></span>[[#_Ref132914048|[17]]]. Building upon this research, Zhang et al. investigated the mechanical response of cylindrical superconductors under flux jumps and analyzed the mechanical response characteristics of superconductors under different conditions <span id='cite-_Ref132914123'></span>[[#_Ref132914123|[18]]]. Wang et al. have explored the mechanical stress issues of high-temperature superconducting ring bulk samples during the PFM process <span id='cite-_Ref132916033'></span>[[#_Ref132916033|[20]]]. |
| − | During the fabrication process of bulk superconductor, the occurrence of small crack and voids is inevitable, which can have a certain impact on the mechanical properties of the bulk superconductor<span id='cite-_Ref135916399'></span>[[#_Ref135916399| | + | During the fabrication process of bulk superconductor, the occurrence of small crack and voids is inevitable, which can have a certain impact on the mechanical properties of the bulk superconductor <span id='cite-_Ref135916399'></span>[[#_Ref135916399|[30]]]. Therefore the study of crack in bulk superconductor is a wide field of scientific research and many researchers have done important work in this field <span id='cite-_Ref144388174'></span>[[#_Ref144388174|[21,22,23,24]]]. Mochizuki ''et al. ''have analyzed the trapped field characteristics and fracture behavior of REBaCuO circular bulk samples during the PFM process <span id='cite-_Ref132916814'></span>[[#_Ref132916814|[25]]]. Takahashi et al. have studied the fracture behavior of EuBaCuO superconducting circular bulk samples under different reinforcing material supports during field cooling magnetization <span id='cite-_Ref132897972'></span>[[#_Ref132897972|[12]]]. Electromagnetic forces lead to stress concentration and crack formation within the superconductor, and the rate and direction of crack propagation depend on factors such as the magnitude and direction of the electromagnetic forces, material parameters, and geometric shape <span id='cite-_Ref135916686'></span>[[#_Ref135916686|[31]]]. Ru et al. conducted numerical simulations to investigate the fracture behavior of bulk superconductor with pre-existing elliptical crack under the influence of electromagnetic forces, delving into the patterns of crack formation and propagation <span id='cite-_Ref135916990'></span>[[#_Ref135916990|[32]]]. |
| − | + | In this paper, the performance of ring bulk superconductor during the PFM magnetization process is analyzed using finite element method based on H-formulation, heat conduction equation, and the mechanical equilibrium equation. The variation in critical current density of the bulk due to variations in magnetic field intensity, temperature, and stress are considered, and the impact of these factors on the results is examined. The analysis results are in good agreement with experimental results. Furthermore, the influence of crack at different locations on the results is analyzed, providing different simulation results for trapped fields and temperatures within the bulk affected by stress and various crack configurations. | |
| − | =2 Numerical model and parameters= | + | ==2. Numerical model and parameters== |
| − | The numerical model in this paper is based on the experimental sample presented by | + | The numerical model in this paper is based on the experimental sample presented by Mochizuki <span id='cite-_Ref132916814'></span>[[#_Ref132916814|[25]]], and the 2D axisymmetric model of the GdBCO ring bulk is adopted for simplification. <span id='cite-_Ref143373672'></span>[[#_Ref143373672|Figure 1]](a) depicts a three-dimensional schematic diagram and a 2D axisymmetric cross-section schematic (not to scale) of the ring GdBCO high-temperature bulk superconductor. The ring is installed in the holder with an aluminum alloy ring (5 mm in width). The lower part of the bulk is a layer of indium sheet (1 mm in thickness), which is connected to the cold head with an initial temperature of 65 K. A uniform critical current is assumed throughout the ring in this study, and the epoxy layer between the aluminum alloy ring and the superconducting ring is disregarded, assuming an ideal connection between them. In the experiment, a pulsed field of <math display="inline">B_{ex}=2.53\mbox{ }T</math> was applied to the bulk, with a rise time of 13 ms and a duration of 200 ms. Hall sensors were positioned at the center of the bulk to measure the trapped field, while a Hall probe was placed 1 mm above the surface of the bulk to generate the distribution of the trapped field <math display="inline">B_z</math> <span id='cite-_Ref132916814'></span>[[#_Ref132916814|[25]]]. High-temperature copper oxide superconductors are relatively brittle, microcrack and defects can be generated in superconductors <span id='cite-_Ref143947786'></span>[[#_Ref143947786|[36]]]. Cracking may occur in superconductors due to large electromagnetic body force <span id='cite-_Ref143947968'></span>[[#_Ref143947968|[37]]]. Furthermore, the crack and defects lead to strong local enhancement of the stress which may limit the application of the superconductor. In order to investigate the impact of crack on the superconducting and mechanical properties of the bulk superconductor, elliptical crack were introduced into the model, while both central crack case and edge crack case are considered, as shown in [[#_Ref143373672|Figures 1]](b) and (c). |
| − | <div class=" | + | <div id="_Ref143373672"></div> |
| − | + | {| class="wikitable" style="margin: 0em auto 0.1em auto;border-collapse: collapse;width:60%;" | |
| + | |-style="background:white;" | ||
| + | |style="text-align: center;padding:10px;"| [[Image:Review_893267287081-image3.png|402px]] | ||
| + | |- | ||
| + | | style="background:#efefef;text-align:left;padding:10px;font-size: 85%;"| '''Figure 1'''. (a) Sketches of the ring GdBCO bulk (left) and two-dimensional axisymmetric model (right). (b)-(c) Two-dimensional axisymmetric modeling of bulk superconductor containing central elliptical crack case and edge semi-elliptical crack case | ||
| + | |} | ||
| − | |||
| − | |||
| − | In this study, the magnetic field and current density were calculated using the H-formulation<span id='cite-_Ref143949294'></span>[[#_Ref143949294| | + | In this study, the magnetic field and current density were calculated using the H-formulation<span id='cite-_Ref143949294'></span>[[#_Ref143949294|[38]]]. The governing equations are given by <span id='cite-_Ref132979450'></span>[[#_Ref132979450|[26]]] |
{| class="formulaSCP" style="width: 100%; text-align: center;" | {| class="formulaSCP" style="width: 100%; text-align: center;" | ||
| Line 52: | Line 56: | ||
|} | |} | ||
| − | + | where <math>\boldsymbol{E}</math>, <math display="inline">\boldsymbol{B}</math> and <math display="inline">\boldsymbol{H}</math> are electric field, magnetic flux density, and magnetic field intensity, respectively. | |
| − | + | ||
The Maxwell's equations, which are required for electromagnetic field calculations, are as follows | The Maxwell's equations, which are required for electromagnetic field calculations, are as follows | ||
| Line 71: | Line 74: | ||
|} | |} | ||
| − | + | where <math>\boldsymbol{J}</math> is the critical current density, and <math>t</math> is the time. The electromagnetic constitutive equations for superconductors are as follows | |
| − | + | ||
{| class="formulaSCP" style="width: 100%; text-align: center;" | {| class="formulaSCP" style="width: 100%; text-align: center;" | ||
| Line 79: | Line 81: | ||
{| style="text-align: center; margin:auto;" | {| style="text-align: center; margin:auto;" | ||
|- | |- | ||
| − | | <span id='ZEqnNum216404'></span> <math>\begin{cases} \boldsymbol{E}=\rho \boldsymbol{J}\\ \boldsymbol{B}={\mu }_0{\mu }_r\boldsymbol{H} \end{cases}</math> | + | | <span id='ZEqnNum216404'></span> <math>\begin{cases} \boldsymbol{E}=\rho \boldsymbol{J} \\ \boldsymbol{B}={\mu }_0{\mu }_r\boldsymbol{H} \end{cases}</math> |
|} | |} | ||
| style="width: 5px;text-align: right;white-space: nowrap;" | (3) | | style="width: 5px;text-align: right;white-space: nowrap;" | (3) | ||
|} | |} | ||
| − | + | where <math>\rho </math> is the equivalent resistivity; the variables <math>{\mu }_0</math> and <math display="inline">{\mu }_r</math> are the Vacuum permeability, and the relative permeability, in this study, a value of <math>{\mu }_r=1</math>. Furthermore, Eq. <span id='cite-ZEqnNum769201'></span>[[#ZEqnNum769201|(1)]] is derived by combining Eqs. <span id='cite-ZEqnNum320282'></span>[[#ZEqnNum320282|(2)]] and <span id='cite-ZEqnNum216404'></span>[[#ZEqnNum216404|(3)]]. To prevent the electric field from becoming excessively large when the current exceeds the critical density in the <math display="inline">\boldsymbol{E-J}</math> model, the modified <math display="inline">\boldsymbol{E-J}</math> model is employed, with an effective resistivity denoted as <span id='cite-_Ref142706874'></span>[[#_Ref142706874|[35]]] | |
| − | + | ||
{| class="formulaSCP" style="width: 100%; text-align: center;" | {| class="formulaSCP" style="width: 100%; text-align: center;" | ||
| Line 92: | Line 93: | ||
{| style="text-align: center; margin:auto;" | {| style="text-align: center; margin:auto;" | ||
|- | |- | ||
| − | | <math> | + | | <math>f(n) = \begin{cases} \displaystyle\frac{{\rho }_{sc}\cdot {\rho }_{normal}}{{\rho }_{sc}+{\rho }_{normal}}, & \mbox{ }T\leq T_c \\ {\rho }_{nomal}, & \mbox{ }T>T_c \end{cases}</math> |
|} | |} | ||
| style="width: 5px;text-align: right;white-space: nowrap;" | (4) | | style="width: 5px;text-align: right;white-space: nowrap;" | (4) | ||
|} | |} | ||
| − | + | where <math>{\rho }_{normal}</math> is the resistivity of the bulk superconductor in the normal state. Among them | |
| − | + | ||
{| class="formulaSCP" style="width: 100%; text-align: center;" | {| class="formulaSCP" style="width: 100%; text-align: center;" | ||
| Line 123: | Line 123: | ||
|} | |} | ||
| − | + | where <math>{\beta }_0=1.32\times {10}^9\mbox{A}/\mbox{m}^2</math> <span id='cite-_Ref132985662'></span>[[#_Ref132985662|[27]]], is the critical current density at <math>B=0,\,T =0</math> is determined; <math>T_c</math> is the critical temperature; <math>B_0</math> is the fitted parameter. <math>J\left(\sigma \right)</math> denotes the effect of stress on the critical current density <span id='cite-_Ref142674225'></span>[[#_Ref142674225|[34]]]. The heat transfer control equation is <span id='cite-_Ref132987311'></span>[[#_Ref132987311|[28]]] | |
| − | + | ||
{| class="formulaSCP" style="width: 100%; text-align: center;" | {| class="formulaSCP" style="width: 100%; text-align: center;" | ||
| Line 131: | Line 130: | ||
{| style="text-align: center; margin:auto;" | {| style="text-align: center; margin:auto;" | ||
|- | |- | ||
| − | | <math>{\rho }_mC\frac{\partial T}{\partial t}=\nabla \cdot \left(\kappa \nabla T\right)+ | + | | <math>{\rho }_mC\frac{\partial T}{\partial t}=\nabla \cdot \left(\kappa \nabla T\right)+Q</math> |
|} | |} | ||
| style="width: 5px;text-align: right;white-space: nowrap;" | (7) | | style="width: 5px;text-align: right;white-space: nowrap;" | (7) | ||
| Line 146: | Line 145: | ||
|} | |} | ||
| + | where <math>\rho </math> is the densities; <math>c</math> is the constant pressure heat capacity; <math>k</math> is the thermal conductivity and <math>Q</math> is the joule heat. The thermal conductivity has different values in the ab plane (<math display="inline">{\kappa }_{ab}</math>) and c plane (<math display="inline">{\kappa }_c</math>). Due to the temperature-dependent specific heat and thermal conductivity of the bulk superconductor, fitting equations obtained from the literature were utilized in our program <span id='cite-_Ref132811980'></span>[[#_Ref132811980|[6]]]. The bulk superconductor was affixed to an indium foil, and a cold head was positioned at the bottom of the indium foil, with the temperature designated as <math display="inline">T_0</math>. Therefore, the heat transfer boundary condition is defined as the fixed temperature of <math display="inline">T_0</math> at the lower edge, while the remaining boundaries are subjected to thermal insulation conditions. | ||
| − | + | In the experiment, the external magnetic field on the bulk sample was applied using external coils. In the simulation, the external magnetic field was imposed at the boundary of the air domain. The applied pulse magnetic field was <span id='cite-_Ref132987503'></span>[[#_Ref132987503|[29]]] | |
| − | + | ||
| − | In the experiment, the external magnetic field on the bulk sample was applied using external coils. In the simulation, the external magnetic field was imposed at the boundary of the air domain. The applied pulse magnetic field was<span id='cite-_Ref132987503'></span>[[#_Ref132987503| | + | |
{| class="formulaSCP" style="width: 100%; text-align: center;" | {| class="formulaSCP" style="width: 100%; text-align: center;" | ||
| Line 156: | Line 154: | ||
{| style="text-align: center; margin:auto;" | {| style="text-align: center; margin:auto;" | ||
|- | |- | ||
| − | | <math>B_{ex}\left(t\right)=B_{max}t/{t_1}exp\left(1- | + | | <math>B_{ex}\left(t\right)=B_{\max}t/{t_1}\exp\left(1- t/{t_1}\right)</math> |
|} | |} | ||
| style="width: 5px;text-align: right;white-space: nowrap;" | (9) | | style="width: 5px;text-align: right;white-space: nowrap;" | (9) | ||
|} | |} | ||
| − | + | where <math>B_{\max}</math> is the peak value of the applied magnetic field and <math>t_1</math> is the rise time of the applied magnetic field. | |
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
In the mechanical calculations, the ring bulk sample is considered as an isotropic linear elastic material. The mechanical equilibrium equation can be expressed as follows | In the mechanical calculations, the ring bulk sample is considered as an isotropic linear elastic material. The mechanical equilibrium equation can be expressed as follows | ||
| Line 242: | Line 168: | ||
{| style="text-align: center; margin:auto;" | {| style="text-align: center; margin:auto;" | ||
|- | |- | ||
| − | | <math>\nabla \cdot \boldsymbol{\sigma }+\boldsymbol{F}_L= | + | | <math>\nabla \cdot \boldsymbol{\sigma }+\boldsymbol{F}_L=0</math> |
|} | |} | ||
| style="width: 5px;text-align: right;white-space: nowrap;" | (10) | | style="width: 5px;text-align: right;white-space: nowrap;" | (10) | ||
| Line 257: | Line 183: | ||
|} | |} | ||
| − | + | where <math>\boldsymbol{F}_L</math> is the Lorentz force; <math>\overline{\rho }</math> is the mass density. In the study, it is assumed that the transient behavior of the structure is quasi-static and that the second-order time derivative of the displacement variable <math display="inline">u</math>. The inertia terms <math display="inline">\rho \frac{\mbox{d}^2u}{\mbox{d}t^2}</math> are zero. Due to the occurrence of the Lorentz force during the magnetization process of the high-temperature superconducting ring bulk sample in a pulsed field, the influence of the stress distribution caused by the Lorentz force is considered in the mechanical calculations of this study. It is assumed that the bulk superconductor is fixed on a base, thus imposing a mechanical boundary condition of zero displacement at the lower edge. The stress-strain relationship in the ring bulk sample is given by | |
| − | + | ||
{| class="formulaSCP" style="width: 100%; text-align: center;" | {| class="formulaSCP" style="width: 100%; text-align: center;" | ||
| Line 271: | Line 196: | ||
{\gamma }_{zr} | {\gamma }_{zr} | ||
\end{array}\right]=\left[\begin{array}{cccc} | \end{array}\right]=\left[\begin{array}{cccc} | ||
| − | \frac{1}{E} & -\frac{\upsilon }{E} & -\frac{\upsilon }{E} & 0\\ | + | \displaystyle\frac{1}{E} & -\displaystyle\frac{\upsilon }{E} & -\displaystyle\frac{\upsilon }{E} & 0\\ |
| − | -\frac{\upsilon }{E} & \frac{1}{E} & -\frac{\upsilon }{E} & 0\\ | + | -\displaystyle\frac{\upsilon }{E} & \displaystyle\frac{1}{E} & -\displaystyle\frac{\upsilon }{E} & 0\\ |
| − | -\frac{\upsilon }{E} & -\frac{\upsilon }{E} & \frac{1}{E} & 0\\ | + | -\displaystyle\frac{\upsilon }{E} & -\displaystyle\frac{\upsilon }{E} & \displaystyle\frac{1}{E} & 0\\ |
0 & 0 & 0 & \frac{1}{G} | 0 & 0 & 0 & \frac{1}{G} | ||
\end{array}\right]\left[\begin{array}{c} | \end{array}\right]\left[\begin{array}{c} | ||
| Line 285: | Line 210: | ||
|} | |} | ||
| − | + | where <math display="inline">\upsilon </math> is the Poisson's ratio and <math display="inline">E</math> is the Young's modulus. | |
| − | + | ||
The simulation and modeling of the aforementioned aspects were conducted using the finite element method. The parameter values mentioned earlier but not provided were provided in <span id='cite-_Ref143373393'></span>[[#_Ref143373393|Table 1]]. | The simulation and modeling of the aforementioned aspects were conducted using the finite element method. The parameter values mentioned earlier but not provided were provided in <span id='cite-_Ref143373393'></span>[[#_Ref143373393|Table 1]]. | ||
| − | = | + | <div class="center" style="font-size: 75%;">'''Table 1'''. Parameters in the simulation</div> |
| − | '' | + | <div id='_Ref143373393'></div> |
| + | {| class="wikitable" style="margin: 1em auto 0.1em auto;border-collapse: collapse;font-size:85%;width:auto;" | ||
| + | |-style="text-align:center" | ||
| + | ! Parameter !! style="text-align: left;"|Description !! Value | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">c</math> | ||
| + | | style="text-align: left;vertical-align: top;"|Specific heat of the ring bulk | ||
| + | | style="text-align: center;vertical-align: top;"|<span id='cite-_Ref132811980'></span>[[#_Ref132811980|[6]]] | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">c_{in}</math> | ||
| + | | style="text-align: left;vertical-align: top;"|Thermal conductivity of the indium | ||
| + | | style="text-align: center;vertical-align: top;"|<span id='cite-_Ref132901240'></span>[[#_Ref132901240|[15]]] | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">c_{al}</math> | ||
| + | | style="text-align: left;vertical-align: top;"|Specific heat of the reinforced ring | ||
| + | | style="text-align: center;vertical-align: top;"|<span id='cite-_Ref136003622'></span>[[#_Ref136003622|[33]]] | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">k_{ab}</math> | ||
| + | | style="text-align: left;vertical-align: top;"|Thermal conductivity of the bulk along the ab plane | ||
| + | | style="text-align: center;vertical-align: top;"|<span id='cite-_Ref132811980'></span>[[#_Ref132811980|[6]]] | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">k_c</math> | ||
| + | | style="text-align: left;vertical-align: top;"|Thermal conductivity of the bulk along the c plane | ||
| + | | style="text-align: center;vertical-align: top;"|<span id='cite-_Ref132811980'></span>[[#_Ref132811980|[6]]] | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">k_{in}</math> | ||
| + | | style="text-align: left;vertical-align: top;"|Thermal conductivity of the indium | ||
| + | | style="text-align: center;vertical-align: top;"|<span id='cite-_Ref132901240'></span>[[#_Ref132901240|[15]]] | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">k_{al}</math> | ||
| + | | style="text-align: left;vertical-align: top;"|Thermal conductivity of the reinforced ring | ||
| + | | style="text-align: center;vertical-align: top;"|<span id='cite-_Ref136003622'></span>[[#_Ref136003622|[33]]] | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">\rho </math> | ||
| + | | style="text-align: left;vertical-align: top;"|Density of the ring bulk | ||
| + | | style="text-align: center;vertical-align: top;width: 23%;"|<math display="inline">5900\,</math> kg/m<math display="inline">^3</math> | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">E</math> | ||
| + | | style="text-align: left;vertical-align: top;"|Young modulus of the ring bulk | ||
| + | | style="text-align: center;vertical-align: top;width: 23%;"|<math display="inline">\mbox{103 }</math> GPa | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">\upsilon </math> | ||
| + | | style="text-align: left;vertical-align: top;"|Poisson ratio of the ring bulk | ||
| + | | style="text-align: center;vertical-align: top;width: 23%;"|<math display="inline">0.3</math> | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">\alpha </math> | ||
| + | | style="text-align: left;vertical-align: top;"|Thermal expansivity of the ring bulk | ||
| + | | style="text-align: center;vertical-align: top;width: 23%;"|<math display="inline">1\times {10}^{-5}{\mbox{ K}}^{-1}</math> | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">E_{al}</math> | ||
| + | | style="text-align: left;vertical-align: top;"|Young modulus of the reinforced ring | ||
| + | | style="text-align: center;vertical-align: top;width: 23%;"|<math display="inline">78</math> GPa | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">{\upsilon }_{al}</math> | ||
| + | | style="text-align: left;vertical-align: top;"|Poisson ratio of the reinforced ring | ||
| + | | style="text-align: center;vertical-align: top;width: 23%;"|<math display="inline">0.34</math> | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">{\alpha }_{al}</math> | ||
| + | | style="text-align: left;vertical-align: top;"|Thermal expansivity of the reinforced ring | ||
| + | | style="text-align: center;vertical-align: top;width: 23%;"|<math display="inline">1.48\times {10}^{-5}{\mbox{ K}}^{-1}</math> | ||
| + | |- | ||
| + | | style="text-align: center;vertical-align: top;width: 14%;"|<math display="inline">B_0</math> | ||
| + | | style="text-align: left;vertical-align: top;"|Fitting parameters | ||
| + | | style="text-align: center;vertical-align: top;width: 23%;"|<math display="inline">1.3\mbox{ T}</math> | ||
| + | |} | ||
| − | + | ==3. Results and discussions== | |
| − | + | ===3. 1 Verification of results=== | |
| − | + | ||
| − | + | To ensure the accuracy of the simulation results, a comparative validation was conducted with experimental data <span id='cite-_Ref132916814'></span>[[#_Ref132916814|[25]]]. The validation involved a ring bulk superconductor with an inner diameter of 36 mm and an outer diameter of 30 mm, which was reinforced with a 5 mm thick aluminum alloy and affixed to a 1 mm thick indium foil. The ambient temperature during the experiment was maintained at 65 K. <span id='cite-_Ref143373643'></span>[[#_Ref143373643|Figure 2]] shows the trapped field at the center of the ring superconductor obtained from both the numerical simulation and the experimental measurements. By comparing the numerical simulation results with the experimental data, it was found that the numerical results closely approximated the experimental results, providing evidence of the accuracy of the numerical simulation. | |
| − | + | ||
| − | '' | + | <div id='_Ref143373643'></div> |
| + | {| class="wikitable" style="margin: 0em auto 0.1em auto;border-collapse: collapse;width:45%;" | ||
| + | |-style="background:white;" | ||
| + | |style="text-align: center;padding:10px;"| [[Image:Review_893267287081-image72.png|320px]] | ||
| + | |- | ||
| + | | style="background:#efefef;text-align:left;padding:10px;font-size: 85%;"| '''Figure 2'''. Comparison of numerical simulation and experimentally obtained trapped fields, where <math display="inline">B_{ex}=2.53\mbox{ T}</math> and <math display="inline">t_1=13\,\mbox{ ms}</math> | ||
| + | |} | ||
| − | |||
| − | + | ===3.2 Calculated results for ring bulk superconductor=== | |
| − | + | ||
| − | + | To analyze the electromagnetic characteristics of the ring bulk superconductor during the PFM process, [[#_Ref143373901|Figure 3]] shows the magnetic field, and current density distributions of the bulk superconductor at different times (5 ms, 13 ms, 20 ms, 80 ms and 150 ms). As shown in the figure, the magnetic field at the ascending stage (0-13 ms), penetrates from the upper and lower ends of the bulk superconductor towards the interior. Simultaneously, a circular current is generated within the bulk, gradually flowing from the external region into the interior. Conversely, reverse currents begin to appear simultaneously from the upper and lower ends of the bulk sample at the descending stage (after 13ms). | |
| − | Figure 3 | + | |
| − | < | + | <div id='_Ref143373901'></div> |
| + | {| class="wikitable" style="margin: 0em auto 0.1em auto;border-collapse: collapse;width:auto;" | ||
| + | |-style="background:white;" | ||
| + | |style="text-align: center;padding:10px;"| [[Image:Review_893267287081-image75.png|500px]] | ||
| + | |- | ||
| + | | style="background:#efefef;text-align:left;padding:10px;font-size: 85%;"| '''Figure 3'''. Magnetic field and current density distribution in the bulk under the PFM process | ||
| + | |} | ||
| − | |||
| − | |||
| − | < | + | <span id='cite-_Ref143374175'></span>[[#_Ref143374175|Figures 4]](a) and (b) depict the distribution of the trapped field and temperature at different times during the magnetization process on the <math display="inline">z=0</math> plane. The observed variation in the trapped field aligns with the findings in Wu et al. <span id='cite-_Ref132901240'></span>[[#_Ref132901240|[15]]] for cylindrical bulk samples. However, the temperature variation differs from that of cylindrical bulk samples. In the simulation results, it could be found that the temperature gradually increases inward from the right side along the radius and then decreases gradually. This phenomenon arises due to the reinforcement of the bulk sample with an aluminum alloy ring, which affects the thermal conduction between the bulk sample and the aluminum alloy, thereby influencing the temperature distribution. |
| − | + | ||
| − | < | + | <div id='_Ref143374175'></div> |
| + | {| class="wikitable" style="margin: 0em auto 0.1em auto;border-collapse: collapse;width:60%;" | ||
| + | |-style="background:white;" | ||
| + | |style="text-align: center;padding:10px;"| [[Image:Review_893267287081-image76.png|540px]] | ||
| + | |- | ||
| + | | style="background:#efefef;text-align:left;padding:10px;font-size: 85%;"| '''Figure 4'''. Trapped field and temperature distribution in the <math display="inline">z=0</math> plane of the bulk at different times under the PFM process | ||
| + | |} | ||
| − | |||
| − | |||
| − | < | + | For study the mechanical response in the PFM process, <span id='cite-_Ref143374303'></span>[[#_Ref143374303|Figures 5]](a) and (b) present the distribution of radial stress on the <math display="inline">z=0</math> plane, corresponding to the ascending stage and descending stage of the external magnetic field, respectively. In ascending stage, compressive stress is generated within the sample when the magnetic field gradually penetrates into the bulk sample. Subsequently, during the descending stage of the external magnetic field, the radial stress undergoes a transition from compression to tension due to the variation in the direction of the current. <span id='cite-_Ref143374303'></span>[[#_Ref143374303|Figures 5]](c) and (d) show the distribution of hoop stress <math display="inline">{\sigma }_{\varphi }</math> on the <math display="inline">z=0</math> plane of the bulk sample during the magnetization process, corresponding to the ascending stage and descending stage, respectively. It can be observed that during the ascending stage, compressive stress exists at the inner edge of the ring bulk superconductor. As the external magnetic field decreases, the hoop stress at the inner edge gradually transitions to tensile stress. The hoop stress experienced by the inner edge of the ring bulk sample is higher than that of the outer edge. Therefore, it can be inferred that the inner edge of the ring bulk superconductor is more prone to crack formation than the outer edge. In the next section, we will introduce pre-existing crack and analyze their electromagnetic and mechanical characteristics separately, while both of central crack case and edge crack case are considered. |
| − | + | ||
| − | '' | + | <div id='_Ref143374303'></div> |
| + | {| class="wikitable" style="margin: 0em auto 0.1em auto;border-collapse: collapse;width:60%;" | ||
| + | |-style="background:white;" | ||
| + | |style="text-align: center;padding:10px;"| [[Image:Review_893267287081-image78.png|482px]] | ||
| + | |- | ||
| + | | style="background:#efefef;text-align:left;padding:10px;font-size: 85%;"| '''Figure 5'''. Distribution of radial and hoop stresses at different moments in the <math display="inline">z=0</math>plane of a bulk under the action of a pulsed magnetic field | ||
| + | |} | ||
| − | In this section, the simulation results are analyzed for the models of blocks containing elliptical center crack and blocks containing semi-elliptical edge crack, respectively The semi-major axis and semi-minor axis of the elliptical are <math display="inline">a=1\mbox{ mm}</math> , <math display="inline">b=\mbox{0}\mbox{.1 mm}</math> , respectively. | + | ===3. 3 Calculated results for ring bulk superconductor with crack=== |
| + | |||
| + | In this section, the simulation results are analyzed for the models of blocks containing elliptical center crack and blocks containing semi-elliptical edge crack, respectively The semi-major axis and semi-minor axis of the elliptical are <math display="inline">a=1\mbox{ mm}</math>, <math display="inline">b=\mbox{0}\mbox{.1 mm}</math>, respectively. | ||
<span id='cite-_Ref143374521'></span>[[#_Ref143374521|Figure 6]] depict the distributions of magnetic field and current density at 13 ms, 80 ms, and 150 ms for the ring bulk sample with a central elliptical crack and the ring bulk sample with a semi-elliptical edge crack case. It can be observed that the presence of both the central crack case and edge crack case has a minor impact on the distribution of the magnetic field. However, the presence of crack changes the geometric structure of the bulk sample, leading to variations in the current distribution. Additionally, the direction of the current density also variations in the vicinity of the crack. | <span id='cite-_Ref143374521'></span>[[#_Ref143374521|Figure 6]] depict the distributions of magnetic field and current density at 13 ms, 80 ms, and 150 ms for the ring bulk sample with a central elliptical crack and the ring bulk sample with a semi-elliptical edge crack case. It can be observed that the presence of both the central crack case and edge crack case has a minor impact on the distribution of the magnetic field. However, the presence of crack changes the geometric structure of the bulk sample, leading to variations in the current distribution. Additionally, the direction of the current density also variations in the vicinity of the crack. | ||
| + | |||
| + | <div id='Ref143374521'></div> | ||
| + | {| class="wikitable" style="margin: 0em auto 0.1em auto;border-collapse: collapse;width:50%;" | ||
| + | |-style="background:white;" | ||
| + | |style="text-align: center;padding:10px;"| [[Image:Review_893267287081-image82.png|500px]] | ||
| + | |- | ||
| + | | style="background:#efefef;text-align:left;padding:10px;font-size: 85%;"| '''Figure 6'''. Distributions of magnetic field and current density in central crack case (left) and edge crack case (right) | ||
| + | |} | ||
| + | |||
The temperature of the bulk sample gradually increases as the external magnetic field rises. However, the presence of crack also introduces certain variations to the temperature rise trend. As shown in <span id='cite-_Ref143374608'></span>[[#_Ref143374608|Figure 7]], when there are without crack in the bulk sample, the temperature undergoes a sudden jump during the descending stage. The central crack case has a more gradual temperature variation than edge crack case. However, in edge crack case, the temperature experiences two jumps during the descending stage, with a larger amplitude and higher jump temperature. This phenomenon indicates that the presence of edge crack case, will change the direction of the current around the crack. The current concentrated on the region near the crack, resulting in larger electric fields. Due to the strong nonlinearity <math display="inline">\boldsymbol{E-J}</math> relationship of the bulk superconductor, the Joule heat will rise and leads to significant local temperature increase. | The temperature of the bulk sample gradually increases as the external magnetic field rises. However, the presence of crack also introduces certain variations to the temperature rise trend. As shown in <span id='cite-_Ref143374608'></span>[[#_Ref143374608|Figure 7]], when there are without crack in the bulk sample, the temperature undergoes a sudden jump during the descending stage. The central crack case has a more gradual temperature variation than edge crack case. However, in edge crack case, the temperature experiences two jumps during the descending stage, with a larger amplitude and higher jump temperature. This phenomenon indicates that the presence of edge crack case, will change the direction of the current around the crack. The current concentrated on the region near the crack, resulting in larger electric fields. Due to the strong nonlinearity <math display="inline">\boldsymbol{E-J}</math> relationship of the bulk superconductor, the Joule heat will rise and leads to significant local temperature increase. | ||
| − | <div class=" | + | <div id='Ref143374608'></div> |
| − | + | {| class="wikitable" style="margin: 0em auto 0.1em auto;border-collapse: collapse;width:50%;" | |
| + | |-style="background:white;" | ||
| + | |style="text-align: center;padding:10px;"| [[Image:Review_893267287081-image83.png|324px]] | ||
| + | |- | ||
| + | | style="background:#efefef;text-align:left;padding:10px;font-size: 85%;"| '''Figure 7'''. Maximum temperature of the bulk as a function of time in the presence of a pulsed magnetic field | ||
| + | |} | ||
| − | |||
| − | |||
| − | < | + | <span id='cite-_Ref143374695'></span>[[#_Ref143374695|Figure 8]] show the distributions of circumferential and radial stress at 13 ms, 80 ms, and 150 ms for the ring bulk sample with a central elliptical crack and the ring bulk sample with semi-elliptical edge crack case. It can be observed that stress concentration occurs at the crack tips, resulting from the alteration of the bulk sample's geometric shape due to the presence of crack. The crack lead to the stress concentration effect. And this stress concentration phenomenon also leads to the non-uniformity of the critical current density, thereby affecting the distribution of temperature. |
| − | + | ||
| − | <div id= | + | <div id='Ref143374695'></div> |
| − | Figure | + | {| class="wikitable" style="margin: 0em auto 0.1em auto;border-collapse: collapse;width:auto;" |
| + | |-style="background:white;" | ||
| + | |style="text-align: center;padding:10px;"| [[Image:Review_893267287081-image84.png|550px]] | ||
| + | |- | ||
| + | | style="background:#efefef;text-align:left;padding:10px;font-size: 85%;"| '''Figure 8'''. Stress distributions clouds for bulk containing central crack case (left) and edge crack case (right) | ||
| + | |} | ||
| − | |||
| − | |||
| − | < | + | <span id='cite-_Ref143374808'></span>[[#_Ref143374808|Figures 9]](a) and (b) depict the distribution of radial and hoop stress on the <math display="inline">z=0</math> plane at 13 ms and 50 ms, respectively, for the bulk sample without crack, with a central crack case, and with edge crack case. As shown in [[#_Ref143374808|Figure 9]], both radial and hoop stress experience a significant increase at the crack tips, indicating stress concentration. However, the stress values at the crack tips exhibit a more pronounced increase due to the presence of crack. At 13 ms, the stress concentration caused by edge crack case is more obvious than central crack case. The maximum radial stress on the <math display="inline">z=0</math> plane for the bulk sample without crack is <math display="inline">-20.7\,\mbox{MPa}</math>, while for the bulk sample with a central crack case, it is <math display="inline">-36.1\,\mbox{MPa}</math>, representing a 74% increase compared to the crack-free bulk sample. The bulk sample with edge crack case exhibits a maximum hoop stress on the <math display="inline">z=0</math> plane of <math display="inline">37.1\mbox{MPa}</math>, representing a 79% increase compared to the crack-free bulk sample. At 50 ms, the stress concentration caused by central crack case becomes more pronounced than that caused by edge crack case. However, overall, edge crack case can lead to larger stress concentrations than central crack case. |
| − | + | ||
| − | < | + | <div id='Ref143374808'></div> |
| + | {| class="wikitable" style="margin: 0em auto 0.1em auto;border-collapse: collapse;width:60%;" | ||
| + | |-style="background:white;" | ||
| + | |style="text-align: center;padding:10px;"| [[Image:Review_893267287081-image88.png|494px]] | ||
| + | |- | ||
| + | | style="background:#efefef;text-align:left;padding:10px;font-size: 85%;"| '''Figure 9'''. Distribution of radial and hoop stresses without crack and with central case and edge crack case at different times in <math display="inline">z=0</math> plane | ||
| + | |} | ||
| − | |||
The size of the crack length also has a certain influence on the maximum stresses. In <span id='cite-_Ref143374903'></span>[[#_Ref143374903|Figure 10]], we analyze the variation in the maximum radial and hoop stresses among bulk samples with different edge crack lengths case. It can be observed from the figure that both radial and hoop stresses increase with the increase in crack length, with the hoop stress exhibiting a more pronounced trend. This phenomenon occurs because as the crack length increases, the stress concentration effect becomes more prominent. When the crack is shorter, the sharpness at the crack tip is lower. However, as the crack length grows, the sharpness at the crack tip increases, and the region of high stress expands accordingly, leading to higher stresses in the sample. | The size of the crack length also has a certain influence on the maximum stresses. In <span id='cite-_Ref143374903'></span>[[#_Ref143374903|Figure 10]], we analyze the variation in the maximum radial and hoop stresses among bulk samples with different edge crack lengths case. It can be observed from the figure that both radial and hoop stresses increase with the increase in crack length, with the hoop stress exhibiting a more pronounced trend. This phenomenon occurs because as the crack length increases, the stress concentration effect becomes more prominent. When the crack is shorter, the sharpness at the crack tip is lower. However, as the crack length grows, the sharpness at the crack tip increases, and the region of high stress expands accordingly, leading to higher stresses in the sample. | ||
| − | <div | + | <div id='Ref143374903'></div> |
| − | + | {| class="wikitable" style="margin: 0em auto 0.1em auto;border-collapse: collapse;width:60%;" | |
| − | + | |-style="background:white;" | |
| − | + | |style="text-align: center;padding:10px;"| [[Image:Review_893267287081-image89.png|358px]] | |
| − | + | |- | |
| − | + | | style="background:#efefef;text-align:left;padding:10px;font-size: 85%;"| '''Figure 10'''. Maximum radial and hoop stresses during magnetization of bulk containing edge crack case of different lengths | |
| − | + | |} | |
| − | + | ||
| − | + | ||
| − | + | ||
| − | Figure 10 Maximum radial and hoop stresses during magnetization of bulk containing edge crack case of different lengths | + | |
| − | =4 Conclusions= | + | ==4. Conclusions== |
In this study, the distribution of electromagnetic fields, temperature, and stress in ring bulk superconductor was simulated and analyzed by employing the H-formulation, heat conduction equation, and mechanical equilibrium equation. The coupled equations of force-electric-magnetic-thermal multi-field were solved to obtain the simulation results, which exhibited a good agreement with the experimental findings. | In this study, the distribution of electromagnetic fields, temperature, and stress in ring bulk superconductor was simulated and analyzed by employing the H-formulation, heat conduction equation, and mechanical equilibrium equation. The coupled equations of force-electric-magnetic-thermal multi-field were solved to obtain the simulation results, which exhibited a good agreement with the experimental findings. | ||
| Line 385: | Line 402: | ||
==References== | ==References== | ||
| + | <div class="auto" style="text-align: left;width: auto; margin-left: auto; margin-right: auto;font-size: 85%;"> | ||
| − | <span id='_Ref132794187'></span | + | <span id='_Ref132794187'></span>[[#cite-_Ref132794187|[1]]] Murakami M. Processing and applications of bulk RE-Ba-Cu-O superconductors. Int J. Appl. Ceramic. Tech., 4:225–241, 2007. |
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | <span id=' | + | <span id='_Ref132794189'></span>[[#cite-_Ref132794189|[2]]] Hull J.R., Murakami M. Applications of bulk high-temperature superconductors. Proc. IEEE., 92:1705–1718, 2004. |
| − | <span id=' | + | <span id='_Ref132794191'></span>[[#cite-_Ref132794191|[3]]] Zou J., Ainslie M.D., Fujishiro H., et al. Numerical modelling and comparison of MgB2 bulks fabricated by HIP and infiltration growth. Supercond. Sci. Technol., 28, 075009, 2015. |
| − | <span id=' | + | <span id='_Ref132794192'></span>[[#cite-_Ref132794192|[4]]] Yokoyama K., Oka T., Okada H., et al. Solid-liquid magnetic separation using bulk superconducting magnets. IEEE Trans. Appl. Supercond., 13:1592–1595, 2003. |
| − | <span id=' | + | <span id='_Ref132811978'></span>[[#cite-_Ref132811978|[5]]] Ainslie M.D., Fujishiro H., Ujiie T., et al. Modelling and comparison of trapped fields in (RE)BCO bulk superconductors for activation using pulsed field magnetization. Supercond. Sci. Technol., 27, 065008, 2014. |
| − | <span id=' | + | <span id='_Ref132811980'></span>[[#cite-_Ref132811980|[6]]] Ainslie M.D., Fujishiro H. Modelling of bulk superconductor magnetization. Supercond. Sci. Technol., 28, 053002, 2015. |
| − | <span id=' | + | <span id='_Ref132811982'></span>[[#cite-_Ref132811982|[7]]] Ainslie M.D., Fujishiro H., Mochizuki H., et al. Enhanced trapped field performance of bulk high-temperature superconductors using split coil, pulsed field magnetization with an iron yoke. Supercond. Sci. Technol., 29, 074003, 2016. |
| − | <span id=' | + | <span id='_Ref132811983'></span>[[#cite-_Ref132811983|[8]]] Zhou D., Shi Y., Dennis A.R., et al. Flux jumps in ring-shaped and assembled bulk superconductors during pulsed field magnetization. Supercond. Sci. Technol., 33, 034001, 2020. |
| − | <span id=' | + | <span id='_Ref132811985'></span>[[#cite-_Ref132811985|[9]]] Zhou D., Ainslie M.D., Srpčič J., et al. Exploiting flux jumps for pulsed field magnetisation. Supercond. Sci. Technol., 31, 105005, 2018. |
| − | <span id=' | + | <span id='_Ref132814132'></span><span id='_Hlk139036325'>[[#cite-_Hlk139036325|[10]]] Fujishiro H., Tateiwa T., et al. Higher trapped field over 5T on HTSC bulk by modified pulse field magnetizing. Physica C: Superconductivity and its Applications, 445–448:334-338, 2006. |
| − | <span id=' | + | <span id='_Ref132897971'><span id='_Hlk139038521'></span>[[#cite-_Hlk139038521|[11]]] Takahashi K., Fujishiro H., et al. Fracture behavior analysis of EuBaCuO superconducting ring bulk reinforced by a stainless steel ring during field-cooled magnetization. Supercond. Sci. Technol., 30(11), 115006, 2017. |
| − | <span id=' | + | <span id='_Ref132897972'></span>[[#cite-_Ref132897972|[12]]] Takahashi K., Namba S., et al. Thermal and magnetic strain measurements on a REBaCuO ring bulk reinforced by a metal ring during field-cooled magnetization. Supercond. Sci. Technol., 32(1), 015007, 2019. |
| − | <span id=' | + | <span id='_Ref144388679'></span>[[#cite-_Ref144388679|[13]]] Ainslie M.D., Huang K.Y., Fujishiro H., et al. Numerical modelling of mechanical stresses in bulk superconductor magnets with and without mechanical reinforcement. Superconductor Science and Technology, 32(3), 034002, 2019. |
| − | <span id=' | + | <span id='_Ref144388911'></span>[[#cite-_Ref144388911|[14]]] Li R., Fang J., Liao X., et al. Electromagnetic and thermal properties of HTS bulk combined with different ferromagnetic structures under pulsed field magnetization. IEEE Transactions on Applied Superconductivity, 33(6):1-6, 2023. |
| − | <span id=' | + | <span id='_Ref132901240'></span><span id='_Hlk139048497'>[[#cite-_Hlk139048497|[15]]] Wu H., Yong H., et al. Stress analysis in high-temperature superconductors under pulsed field magnetization. Superconductor Science and Technology, 31(4), 045008, 2018. |
| − | <span id=' | + | <span id='_Ref132901719'><span id='_Hlk139048580'></span>[[#cite-_Hlk139048580|[16]]] Wu H., Yong H., et al. Analysis of mechanical behavior in inhomogeneous high temperature superconductors under pulsed field magnetization. Superconductor Science and Technology, 33(12), 124002, 2020. |
| − | <span id=' | + | <span id='_Ref132914048'>[[#cite-_Ref132914048|[17]]] Xia J., Li M., Zhou Y. Numerical investigations on the characteristics of thermomagnetic instability in MgB2 bulks. Superconductor Science and Technology, 30(7), 075004, 2017. |
| − | <span id=' | + | <span id='_Ref132914123'></span>[[#cite-_Ref132914123|[18]]] Zhang W., Xia J., Yong H., et al. Mechanical response induced by flux jump in a cylindrical superconductor. AIP Advances, 10(2), 025021, 2020. |
| − | <span id=' | + | <span id='_Ref132914481'>[[#cite-_Ref132914481|[19]]] Ainslie M.D., Fujishiro H. Modelling of bulk superconductor magnetization. Superconductor Science and Technology, 28(5), 053002, 2015. |
| − | <span id=' | + | <span id='_Ref132916033'></span>[[#cite-_Ref132916033|[20]]] Wang L., Wu H.W., Yong H.D. Mechanical stress in high-temperature superconducting ring-shaped bulk during the pulsed-field magnetization. Science China Technological Sciences, 66:574–585, 2023. |
| − | <span id=' | + | <span id='_Ref144388174'></span>[[#cite-_Ref144388174|[21]]] Chen H., Yong H., Zhou Y. XFEM analysis of the fracture behavior of bulk superconductor in high magnetic field. Journal of Applied Physics, 125(10), 103901, 2019. |
| − | <span id=' | + | <span id='_Ref144388240'></span>[[#cite-_Ref144388240|[22]]] Chen H., Yong H., Zhou Y. Crack detection in bulk superconductor using Genetic Algorithm. Engineering Fracture Mechanics, 265, 108372, 2022. |
| − | <span id=' | + | <span id='_Ref144388367'></span>[[#cite-_Ref144388367|[23]]] Shen H., Ru Y., Wu H., et al. Three-dimensional peridynamic modeling of crack initiation and propagation in bulk superconductor during field cooling magnetization. Superconductor Science and Technology, 34(8), 085020, 2021. |
| − | <span id=' | + | <span id='_Ref144388459'></span>[[#cite-_Ref144388459|[24]]] Ru Y., Yong H., Zhou Y. Numerical simulation of dynamic fracture behavior in bulk superconductors with an electromagnetic-thermal model. Superconductor Science and Technology, 32(7), 074001, 2019. |
| − | <span id=' | + | <span id='_Ref132916814'></span>[[#cite-_Ref132916814|[25]]] Mochizuki H., Fujishiro H., Naito T., et al. Trapped field characteristics and fracture behavior of REBaCuO bulk ring during pulsed field magnetization. IEEE Transactions on Applied Superconductivity, 26(4):1-5, 2015. |
| − | <span id=' | + | <span id='_Ref132979450'></span>[[#cite-_Ref132979450|[26]]] Ainslie M.D., Srpcic J., Zhou D., et al. Toward optimization of multipulse, pulsed field magnetization of bulk high-temperature superconductors. IEEE Trans. Appl. Supercond., 28(4):1–7, 2018. |
| − | <span id=' | + | <span id='_Ref132985662'></span>[[#cite-_Ref132985662|[27]]] Fujishiro H., Naito T. Simulation of temperature and magnetic field distribution in bulk superconductor during pulsed field magnetization. Supercond. Sci. Technol., 23(10), 105021, 2010. |
| − | <span id=' | + | <span id='_Ref132987311'></span>[[#cite-_Ref132987311|[28]]] Ainslie M.D., Zhou D., Fujishiro H., et al. Flux jump-assisted pulsed field magnetisation of high-Jc bulk high-temperature superconductors. Supercond. Sci. Technol., 29, 124004, 2016. |
| − | <span id=' | + | <span id='_Ref132987503'></span>[[#cite-_Ref132987503|[29]]] Fujishiro H., Naito T, Furuta D. Analysis of temperature and magnetic field distribution in bulk superconductor during pulsed field magnetization. IEEE Trans. Appl. Supercond., 21:2723–2726, 2011. |
| − | <span id=' | + | <span id='_Ref135916399'></span>[[#cite-_Ref135916399|[30]]] Diko P. Cracking in melt-processed RE-Ba-Cu-O superconductors. Superconductor Science and Technology, 11(1):68-72, 1998. |
| − | <span id=' | + | <span id='_Ref135916686'></span>[[#cite-_Ref135916686|[31]]] Zhou Y.H., Yong H.D. Crack problem for a long rectangular slab of superconductor under an electromagnetic force. Physical Review B, 76(9), 094523, 2007. |
| − | <span id=' | + | <span id='_Ref135916990'></span>[[#cite-_Ref135916990|[32]]] Ru Y., Yong H., Zhou Y. Fracture analysis of bulk superconductors under electromagnetic force. Engineering Fracture Mechanics, 199:257-273, 2018. |
| − | <span id='_Ref136003622'></span | + | <span id='_Ref136003622'></span>[[#cite-_Ref136003622|[33]]] Callister W., Rethwisch D. Material science and engineering - An introduction. John Wiley & Sons, pp. 990, 2007. |
| − | <span id='_Ref142674225'></span | + | <span id='_Ref142674225'></span>[[#cite-_Ref142674225|[34]]] van der Laan D.C., Ekin J.W. Large intrinsic effect of axial strain on the critical current of high-temperature superconductors for electric power applications. Applied Physics Letters, 90(5), 052506, 2007. |
| − | <span id='_Ref142706874'></span | + | <span id='_Ref142706874'></span>[[#cite-_Ref142706874|[35]]] Duron J., Grilli F., Dutoit B., et al. Modelling the E–J relation of high-Tc superconductors in an arbitrary current range. Physica C: Superconductivity, 401(1-4):231-235, 2004. |
| − | <span id='_Ref143947786'></span | + | <span id='_Ref143947786'></span>[[#cite-_Ref143947786|[36]]] Diko P., Krabbes G. Macro-cracking in melt-grown YBaCuO superconductor induced by surface oxygenation. Superconductor Science and Technology, 16(1):90-93, 2002. |
| − | <span id='_Ref143947968'></span | + | <span id='_Ref143947968'></span>[[#cite-_Ref143947968|[37]]] Ren Y., Weinstein R., Liu J., et al. Damage caused by magnetic pressure at high trapped field in quasi-permanent magnets composed of melt-textured Y-Ba-Cu-O superconductor. Physica C: Superconductivity, 251(1-2):15-26, 1995. |
| − | <span id='_Ref143949294'></span | + | <span id='_Ref143949294'></span>[[#cite-_Ref143949294|[38]]] Hong Z., Campbell A.M., Coombs T.A. Numerical solution of critical state in superconductivity by finite element software. Supercond. Sci. Technol., 19(12):1246-1252, 2006. |
Latest revision as of 16:27, 11 January 2024
Abstract
High-temperature bulk superconductors are widely used in various superconducting devices due to their high critical current density, which enables them to trap high magnetic fields. However, the critical current density of bulk superconductors is influenced by magnetic field, temperature and stress, and both increase of stress and temperature can lead to a decrease in the critical current density. And the influence of magnetic field, current, stress are interactional. In this paper, the multi-field coupled analysis is employed to investigate the electromagnetic and mechanical behavior on bulk superconductor during pulsed field magnetization. The simulation results were compared with the present experimental results. The results showed a good agreement between the simulation and experimental results, further validating the reliability of the numerical simulation method. The magnetic field, current, temperature, and stress distribution and variation within the bulk sample during the pulsed magnetization process were calculated and analyzed. Additionally, the influence of the central crack and edge crack in the bulk superconductor was investigated. The results indicate that the region near the crack experiences a variation in current flow direction, resulting in local temperature rise and significant electromagnetic forces. The tip of the crack exhibits stress concentration, leading to a decrease in critical current density. Bulk supercondutor with edge crack case exhibits greater stress concentration compared to those with central crack case. The impact of crack length on maximum stress was analyzed, revealing that as the crack length increases, both the maximum hoop stress and radial stress within the bulk sample rise.
Keywords:Bulk superconductor, multi-field coupled analysis, critical current density, finite element method, crack
1. Introduction
Due to their high critical current density and ability to trapped higher magnetic fields, high-temperature bulk superconductors have found widespread applications in various superconducting devices [1,2,3,4]. There are three magnetization methods for bulk superconductor: field cooling (FC), zero-field cooling (ZFC), and pulse field magnetization (PFM). Although FC and ZFC can achieve higher trapped fields, they suffer from drawbacks such as long loading times, high energy losses, and the requirement of expensive equipment [5,6,7,8,9]. In contrast, the advantages of PFM are simplicity, convenience, and cost-effectiveness. It features extremely short loading times and low energy losses, while imposing minimal requirements on the equipment. However, the limitations of PFM are the rapid loading rate leading to significant temperature rises, flux jumps and making it difficult to achieve high trapped fields [10].
Due to the weak mechanical properties of bulk superconductor and the large Lorentz force in magnetization, the mechanical behavior is important in the practical application. The bulk superconductors are sometimes reinforced externally with metals to improve their mechanical performance and prevent fractures [11,12,13,14]. Ainslie and Fujishiro addressed the modeling issues of magnetizing bulk superconductors and provided a finite element method to assess and predict their magnetization characteristics [19]. In 2018, Wu et al. investigated the stress distribution in high-temperature superconductors under PFM magnetization process and proposed methods to mitigate stress [15]. Subsequently, they studied the mechanical behavior of non-uniform high-temperature superconductors under PFM magnetization, analyzing the potential for fracture and damage due to non-uniform distributions and their impacts on performance and lifespan [16]. Xia et al. studied the characteristics of thermomagnetic instability in MgB2 bulk samples and provided numerical simulation methods to evaluate and predict their performance [17]. Building upon this research, Zhang et al. investigated the mechanical response of cylindrical superconductors under flux jumps and analyzed the mechanical response characteristics of superconductors under different conditions [18]. Wang et al. have explored the mechanical stress issues of high-temperature superconducting ring bulk samples during the PFM process [20].
During the fabrication process of bulk superconductor, the occurrence of small crack and voids is inevitable, which can have a certain impact on the mechanical properties of the bulk superconductor [30]. Therefore the study of crack in bulk superconductor is a wide field of scientific research and many researchers have done important work in this field [21,22,23,24]. Mochizuki et al. have analyzed the trapped field characteristics and fracture behavior of REBaCuO circular bulk samples during the PFM process [25]. Takahashi et al. have studied the fracture behavior of EuBaCuO superconducting circular bulk samples under different reinforcing material supports during field cooling magnetization [12]. Electromagnetic forces lead to stress concentration and crack formation within the superconductor, and the rate and direction of crack propagation depend on factors such as the magnitude and direction of the electromagnetic forces, material parameters, and geometric shape [31]. Ru et al. conducted numerical simulations to investigate the fracture behavior of bulk superconductor with pre-existing elliptical crack under the influence of electromagnetic forces, delving into the patterns of crack formation and propagation [32].
In this paper, the performance of ring bulk superconductor during the PFM magnetization process is analyzed using finite element method based on H-formulation, heat conduction equation, and the mechanical equilibrium equation. The variation in critical current density of the bulk due to variations in magnetic field intensity, temperature, and stress are considered, and the impact of these factors on the results is examined. The analysis results are in good agreement with experimental results. Furthermore, the influence of crack at different locations on the results is analyzed, providing different simulation results for trapped fields and temperatures within the bulk affected by stress and various crack configurations.
2. Numerical model and parameters
The numerical model in this paper is based on the experimental sample presented by Mochizuki [25], and the 2D axisymmetric model of the GdBCO ring bulk is adopted for simplification. Figure 1(a) depicts a three-dimensional schematic diagram and a 2D axisymmetric cross-section schematic (not to scale) of the ring GdBCO high-temperature bulk superconductor. The ring is installed in the holder with an aluminum alloy ring (5 mm in width). The lower part of the bulk is a layer of indium sheet (1 mm in thickness), which is connected to the cold head with an initial temperature of 65 K. A uniform critical current is assumed throughout the ring in this study, and the epoxy layer between the aluminum alloy ring and the superconducting ring is disregarded, assuming an ideal connection between them. In the experiment, a pulsed field of was applied to the bulk, with a rise time of 13 ms and a duration of 200 ms. Hall sensors were positioned at the center of the bulk to measure the trapped field, while a Hall probe was placed 1 mm above the surface of the bulk to generate the distribution of the trapped field [25]. High-temperature copper oxide superconductors are relatively brittle, microcrack and defects can be generated in superconductors [36]. Cracking may occur in superconductors due to large electromagnetic body force [37]. Furthermore, the crack and defects lead to strong local enhancement of the stress which may limit the application of the superconductor. In order to investigate the impact of crack on the superconducting and mechanical properties of the bulk superconductor, elliptical crack were introduced into the model, while both central crack case and edge crack case are considered, as shown in Figures 1(b) and (c).
In this study, the magnetic field and current density were calculated using the H-formulation[38]. The governing equations are given by [26]
|
|
(1) |
where , and are electric field, magnetic flux density, and magnetic field intensity, respectively.
The Maxwell's equations, which are required for electromagnetic field calculations, are as follows
|
|
(2) |
where is the critical current density, and is the time. The electromagnetic constitutive equations for superconductors are as follows
|
|
(3) |
where is the equivalent resistivity; the variables and are the Vacuum permeability, and the relative permeability, in this study, a value of . Furthermore, Eq. (1) is derived by combining Eqs. (2) and (3). To prevent the electric field from becoming excessively large when the current exceeds the critical density in the model, the modified model is employed, with an effective resistivity denoted as [35]
|
|
(4) |
where is the resistivity of the bulk superconductor in the normal state. Among them
|
|
(5) |
The critical current density could be influenced by the magnetic field intensity, temperature, and stress. In this article, the modified Kim model is employed, as follows
|
|
(6) |
where [27], is the critical current density at is determined; is the critical temperature; is the fitted parameter. denotes the effect of stress on the critical current density [34]. The heat transfer control equation is [28]
|
|
(7) |
|
|
(8) |
where is the densities; is the constant pressure heat capacity; is the thermal conductivity and is the joule heat. The thermal conductivity has different values in the ab plane () and c plane (). Due to the temperature-dependent specific heat and thermal conductivity of the bulk superconductor, fitting equations obtained from the literature were utilized in our program [6]. The bulk superconductor was affixed to an indium foil, and a cold head was positioned at the bottom of the indium foil, with the temperature designated as . Therefore, the heat transfer boundary condition is defined as the fixed temperature of at the lower edge, while the remaining boundaries are subjected to thermal insulation conditions.
In the experiment, the external magnetic field on the bulk sample was applied using external coils. In the simulation, the external magnetic field was imposed at the boundary of the air domain. The applied pulse magnetic field was [29]
|
|
(9) |
where is the peak value of the applied magnetic field and is the rise time of the applied magnetic field.
In the mechanical calculations, the ring bulk sample is considered as an isotropic linear elastic material. The mechanical equilibrium equation can be expressed as follows
|
|
(10) |
|
|
(11) |
where is the Lorentz force; is the mass density. In the study, it is assumed that the transient behavior of the structure is quasi-static and that the second-order time derivative of the displacement variable . The inertia terms are zero. Due to the occurrence of the Lorentz force during the magnetization process of the high-temperature superconducting ring bulk sample in a pulsed field, the influence of the stress distribution caused by the Lorentz force is considered in the mechanical calculations of this study. It is assumed that the bulk superconductor is fixed on a base, thus imposing a mechanical boundary condition of zero displacement at the lower edge. The stress-strain relationship in the ring bulk sample is given by
|
|
(12) |
where is the Poisson's ratio and is the Young's modulus.
The simulation and modeling of the aforementioned aspects were conducted using the finite element method. The parameter values mentioned earlier but not provided were provided in Table 1.
| Parameter | Description | Value |
|---|---|---|
| Specific heat of the ring bulk | [6] | |
| Thermal conductivity of the indium | [15] | |
| Specific heat of the reinforced ring | [33] | |
| Thermal conductivity of the bulk along the ab plane | [6] | |
| Thermal conductivity of the bulk along the c plane | [6] | |
| Thermal conductivity of the indium | [15] | |
| Thermal conductivity of the reinforced ring | [33] | |
| Density of the ring bulk | kg/m | |
| Young modulus of the ring bulk | GPa | |
| Poisson ratio of the ring bulk | ||
| Thermal expansivity of the ring bulk | ||
| Young modulus of the reinforced ring | GPa | |
| Poisson ratio of the reinforced ring | ||
| Thermal expansivity of the reinforced ring | ||
| Fitting parameters |
3. Results and discussions
3. 1 Verification of results
To ensure the accuracy of the simulation results, a comparative validation was conducted with experimental data [25]. The validation involved a ring bulk superconductor with an inner diameter of 36 mm and an outer diameter of 30 mm, which was reinforced with a 5 mm thick aluminum alloy and affixed to a 1 mm thick indium foil. The ambient temperature during the experiment was maintained at 65 K. Figure 2 shows the trapped field at the center of the ring superconductor obtained from both the numerical simulation and the experimental measurements. By comparing the numerical simulation results with the experimental data, it was found that the numerical results closely approximated the experimental results, providing evidence of the accuracy of the numerical simulation.

|
| Figure 2. Comparison of numerical simulation and experimentally obtained trapped fields, where and |
3.2 Calculated results for ring bulk superconductor
To analyze the electromagnetic characteristics of the ring bulk superconductor during the PFM process, Figure 3 shows the magnetic field, and current density distributions of the bulk superconductor at different times (5 ms, 13 ms, 20 ms, 80 ms and 150 ms). As shown in the figure, the magnetic field at the ascending stage (0-13 ms), penetrates from the upper and lower ends of the bulk superconductor towards the interior. Simultaneously, a circular current is generated within the bulk, gradually flowing from the external region into the interior. Conversely, reverse currents begin to appear simultaneously from the upper and lower ends of the bulk sample at the descending stage (after 13ms).
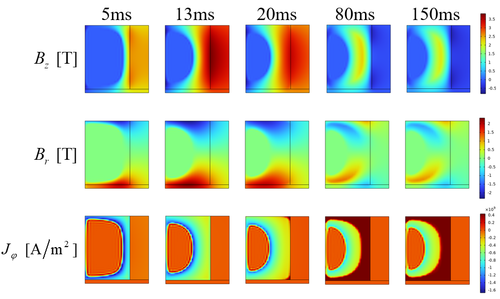
|
| Figure 3. Magnetic field and current density distribution in the bulk under the PFM process |
Figures 4(a) and (b) depict the distribution of the trapped field and temperature at different times during the magnetization process on the plane. The observed variation in the trapped field aligns with the findings in Wu et al. [15] for cylindrical bulk samples. However, the temperature variation differs from that of cylindrical bulk samples. In the simulation results, it could be found that the temperature gradually increases inward from the right side along the radius and then decreases gradually. This phenomenon arises due to the reinforcement of the bulk sample with an aluminum alloy ring, which affects the thermal conduction between the bulk sample and the aluminum alloy, thereby influencing the temperature distribution.
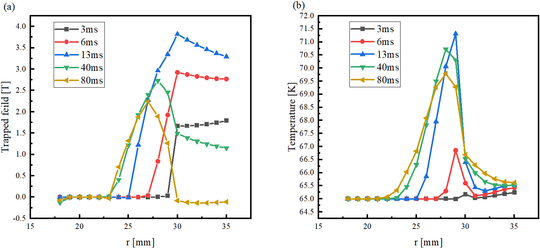
|
| Figure 4. Trapped field and temperature distribution in the plane of the bulk at different times under the PFM process |
For study the mechanical response in the PFM process, Figures 5(a) and (b) present the distribution of radial stress on the plane, corresponding to the ascending stage and descending stage of the external magnetic field, respectively. In ascending stage, compressive stress is generated within the sample when the magnetic field gradually penetrates into the bulk sample. Subsequently, during the descending stage of the external magnetic field, the radial stress undergoes a transition from compression to tension due to the variation in the direction of the current. Figures 5(c) and (d) show the distribution of hoop stress on the plane of the bulk sample during the magnetization process, corresponding to the ascending stage and descending stage, respectively. It can be observed that during the ascending stage, compressive stress exists at the inner edge of the ring bulk superconductor. As the external magnetic field decreases, the hoop stress at the inner edge gradually transitions to tensile stress. The hoop stress experienced by the inner edge of the ring bulk sample is higher than that of the outer edge. Therefore, it can be inferred that the inner edge of the ring bulk superconductor is more prone to crack formation than the outer edge. In the next section, we will introduce pre-existing crack and analyze their electromagnetic and mechanical characteristics separately, while both of central crack case and edge crack case are considered.
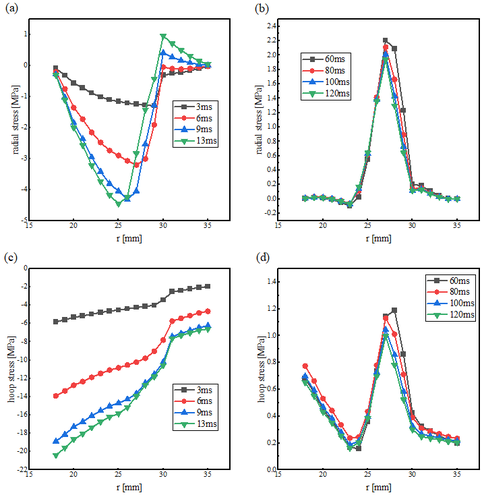
|
| Figure 5. Distribution of radial and hoop stresses at different moments in the plane of a bulk under the action of a pulsed magnetic field |
3. 3 Calculated results for ring bulk superconductor with crack
In this section, the simulation results are analyzed for the models of blocks containing elliptical center crack and blocks containing semi-elliptical edge crack, respectively The semi-major axis and semi-minor axis of the elliptical are , , respectively.
Figure 6 depict the distributions of magnetic field and current density at 13 ms, 80 ms, and 150 ms for the ring bulk sample with a central elliptical crack and the ring bulk sample with a semi-elliptical edge crack case. It can be observed that the presence of both the central crack case and edge crack case has a minor impact on the distribution of the magnetic field. However, the presence of crack changes the geometric structure of the bulk sample, leading to variations in the current distribution. Additionally, the direction of the current density also variations in the vicinity of the crack.
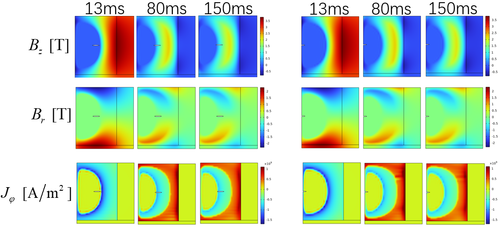
|
| Figure 6. Distributions of magnetic field and current density in central crack case (left) and edge crack case (right) |
The temperature of the bulk sample gradually increases as the external magnetic field rises. However, the presence of crack also introduces certain variations to the temperature rise trend. As shown in Figure 7, when there are without crack in the bulk sample, the temperature undergoes a sudden jump during the descending stage. The central crack case has a more gradual temperature variation than edge crack case. However, in edge crack case, the temperature experiences two jumps during the descending stage, with a larger amplitude and higher jump temperature. This phenomenon indicates that the presence of edge crack case, will change the direction of the current around the crack. The current concentrated on the region near the crack, resulting in larger electric fields. Due to the strong nonlinearity relationship of the bulk superconductor, the Joule heat will rise and leads to significant local temperature increase.
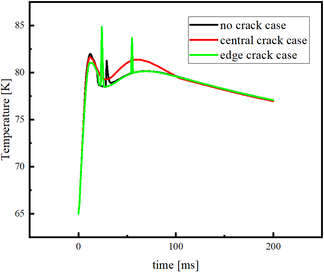
|
| Figure 7. Maximum temperature of the bulk as a function of time in the presence of a pulsed magnetic field |
Figure 8 show the distributions of circumferential and radial stress at 13 ms, 80 ms, and 150 ms for the ring bulk sample with a central elliptical crack and the ring bulk sample with semi-elliptical edge crack case. It can be observed that stress concentration occurs at the crack tips, resulting from the alteration of the bulk sample's geometric shape due to the presence of crack. The crack lead to the stress concentration effect. And this stress concentration phenomenon also leads to the non-uniformity of the critical current density, thereby affecting the distribution of temperature.
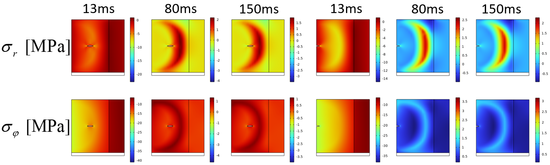
|
| Figure 8. Stress distributions clouds for bulk containing central crack case (left) and edge crack case (right) |
Figures 9(a) and (b) depict the distribution of radial and hoop stress on the plane at 13 ms and 50 ms, respectively, for the bulk sample without crack, with a central crack case, and with edge crack case. As shown in Figure 9, both radial and hoop stress experience a significant increase at the crack tips, indicating stress concentration. However, the stress values at the crack tips exhibit a more pronounced increase due to the presence of crack. At 13 ms, the stress concentration caused by edge crack case is more obvious than central crack case. The maximum radial stress on the plane for the bulk sample without crack is , while for the bulk sample with a central crack case, it is , representing a 74% increase compared to the crack-free bulk sample. The bulk sample with edge crack case exhibits a maximum hoop stress on the plane of , representing a 79% increase compared to the crack-free bulk sample. At 50 ms, the stress concentration caused by central crack case becomes more pronounced than that caused by edge crack case. However, overall, edge crack case can lead to larger stress concentrations than central crack case.
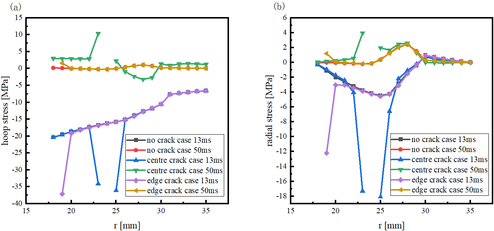
|
| Figure 9. Distribution of radial and hoop stresses without crack and with central case and edge crack case at different times in plane |
The size of the crack length also has a certain influence on the maximum stresses. In Figure 10, we analyze the variation in the maximum radial and hoop stresses among bulk samples with different edge crack lengths case. It can be observed from the figure that both radial and hoop stresses increase with the increase in crack length, with the hoop stress exhibiting a more pronounced trend. This phenomenon occurs because as the crack length increases, the stress concentration effect becomes more prominent. When the crack is shorter, the sharpness at the crack tip is lower. However, as the crack length grows, the sharpness at the crack tip increases, and the region of high stress expands accordingly, leading to higher stresses in the sample.
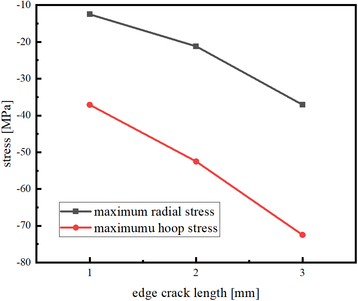
|
| Figure 10. Maximum radial and hoop stresses during magnetization of bulk containing edge crack case of different lengths |
4. Conclusions
In this study, the distribution of electromagnetic fields, temperature, and stress in ring bulk superconductor was simulated and analyzed by employing the H-formulation, heat conduction equation, and mechanical equilibrium equation. The coupled equations of force-electric-magnetic-thermal multi-field were solved to obtain the simulation results, which exhibited a good agreement with the experimental findings.
The research findings indicate that when a pulsed external magnetic field is applied, the magnetic field gradually penetrates into the bulk sample from the upper and lower ends on the right side. Induced currents are generated within the bulk sample, and the direction of these currents variations as the external magnetic field decreases. During the ascending stage of the external magnetic field, compressive stress is generated within the bulk sample. Conversely, as the external magnetic field decreases and the direction of the current variations, tensile stress is produced, leading to an influence on the critical current density of the bulk sample.
Subsequently, simulations were performed on bulk samples with central crack case and edge crack case, respectively, and the distributions of electromagnetic fields, temperature, and stress under PFM were analyzed. The results indicate the occurrence of localized stress concentration due to the presence of crack. Moreover, the crack induce variations in the direction of current and result in localized temperature elevation near the crack vicinity. The maximum radial and hoop stresses within the bulk sample also increase with the length of the crack.
Acknowledgements
The authors gratefully acknowledged the support from the Fujian Natural Science Foundation (2020J05180) and the Scientific Research Foundation of Fujian University of Technology (GY-Z19046). No part of this work has been published or submitted elsewhere. The authors declared that they have no conflicts of interest in this work.
References
[1] Murakami M. Processing and applications of bulk RE-Ba-Cu-O superconductors. Int J. Appl. Ceramic. Tech., 4:225–241, 2007.
[2] Hull J.R., Murakami M. Applications of bulk high-temperature superconductors. Proc. IEEE., 92:1705–1718, 2004.
[3] Zou J., Ainslie M.D., Fujishiro H., et al. Numerical modelling and comparison of MgB2 bulks fabricated by HIP and infiltration growth. Supercond. Sci. Technol., 28, 075009, 2015.
[4] Yokoyama K., Oka T., Okada H., et al. Solid-liquid magnetic separation using bulk superconducting magnets. IEEE Trans. Appl. Supercond., 13:1592–1595, 2003.
[5] Ainslie M.D., Fujishiro H., Ujiie T., et al. Modelling and comparison of trapped fields in (RE)BCO bulk superconductors for activation using pulsed field magnetization. Supercond. Sci. Technol., 27, 065008, 2014.
[6] Ainslie M.D., Fujishiro H. Modelling of bulk superconductor magnetization. Supercond. Sci. Technol., 28, 053002, 2015.
[7] Ainslie M.D., Fujishiro H., Mochizuki H., et al. Enhanced trapped field performance of bulk high-temperature superconductors using split coil, pulsed field magnetization with an iron yoke. Supercond. Sci. Technol., 29, 074003, 2016.
[8] Zhou D., Shi Y., Dennis A.R., et al. Flux jumps in ring-shaped and assembled bulk superconductors during pulsed field magnetization. Supercond. Sci. Technol., 33, 034001, 2020.
[9] Zhou D., Ainslie M.D., Srpčič J., et al. Exploiting flux jumps for pulsed field magnetisation. Supercond. Sci. Technol., 31, 105005, 2018.
[10] Fujishiro H., Tateiwa T., et al. Higher trapped field over 5T on HTSC bulk by modified pulse field magnetizing. Physica C: Superconductivity and its Applications, 445–448:334-338, 2006.
[11] Takahashi K., Fujishiro H., et al. Fracture behavior analysis of EuBaCuO superconducting ring bulk reinforced by a stainless steel ring during field-cooled magnetization. Supercond. Sci. Technol., 30(11), 115006, 2017.
[12] Takahashi K., Namba S., et al. Thermal and magnetic strain measurements on a REBaCuO ring bulk reinforced by a metal ring during field-cooled magnetization. Supercond. Sci. Technol., 32(1), 015007, 2019.
[13] Ainslie M.D., Huang K.Y., Fujishiro H., et al. Numerical modelling of mechanical stresses in bulk superconductor magnets with and without mechanical reinforcement. Superconductor Science and Technology, 32(3), 034002, 2019.
[14] Li R., Fang J., Liao X., et al. Electromagnetic and thermal properties of HTS bulk combined with different ferromagnetic structures under pulsed field magnetization. IEEE Transactions on Applied Superconductivity, 33(6):1-6, 2023.
[15] Wu H., Yong H., et al. Stress analysis in high-temperature superconductors under pulsed field magnetization. Superconductor Science and Technology, 31(4), 045008, 2018.
[16] Wu H., Yong H., et al. Analysis of mechanical behavior in inhomogeneous high temperature superconductors under pulsed field magnetization. Superconductor Science and Technology, 33(12), 124002, 2020.
[17] Xia J., Li M., Zhou Y. Numerical investigations on the characteristics of thermomagnetic instability in MgB2 bulks. Superconductor Science and Technology, 30(7), 075004, 2017.
[18] Zhang W., Xia J., Yong H., et al. Mechanical response induced by flux jump in a cylindrical superconductor. AIP Advances, 10(2), 025021, 2020.
[19] Ainslie M.D., Fujishiro H. Modelling of bulk superconductor magnetization. Superconductor Science and Technology, 28(5), 053002, 2015.
[20] Wang L., Wu H.W., Yong H.D. Mechanical stress in high-temperature superconducting ring-shaped bulk during the pulsed-field magnetization. Science China Technological Sciences, 66:574–585, 2023.
[21] Chen H., Yong H., Zhou Y. XFEM analysis of the fracture behavior of bulk superconductor in high magnetic field. Journal of Applied Physics, 125(10), 103901, 2019.
[22] Chen H., Yong H., Zhou Y. Crack detection in bulk superconductor using Genetic Algorithm. Engineering Fracture Mechanics, 265, 108372, 2022.
[23] Shen H., Ru Y., Wu H., et al. Three-dimensional peridynamic modeling of crack initiation and propagation in bulk superconductor during field cooling magnetization. Superconductor Science and Technology, 34(8), 085020, 2021.
[24] Ru Y., Yong H., Zhou Y. Numerical simulation of dynamic fracture behavior in bulk superconductors with an electromagnetic-thermal model. Superconductor Science and Technology, 32(7), 074001, 2019.
[25] Mochizuki H., Fujishiro H., Naito T., et al. Trapped field characteristics and fracture behavior of REBaCuO bulk ring during pulsed field magnetization. IEEE Transactions on Applied Superconductivity, 26(4):1-5, 2015.
[26] Ainslie M.D., Srpcic J., Zhou D., et al. Toward optimization of multipulse, pulsed field magnetization of bulk high-temperature superconductors. IEEE Trans. Appl. Supercond., 28(4):1–7, 2018.
[27] Fujishiro H., Naito T. Simulation of temperature and magnetic field distribution in bulk superconductor during pulsed field magnetization. Supercond. Sci. Technol., 23(10), 105021, 2010.
[28] Ainslie M.D., Zhou D., Fujishiro H., et al. Flux jump-assisted pulsed field magnetisation of high-Jc bulk high-temperature superconductors. Supercond. Sci. Technol., 29, 124004, 2016.
[29] Fujishiro H., Naito T, Furuta D. Analysis of temperature and magnetic field distribution in bulk superconductor during pulsed field magnetization. IEEE Trans. Appl. Supercond., 21:2723–2726, 2011.
[30] Diko P. Cracking in melt-processed RE-Ba-Cu-O superconductors. Superconductor Science and Technology, 11(1):68-72, 1998.
[31] Zhou Y.H., Yong H.D. Crack problem for a long rectangular slab of superconductor under an electromagnetic force. Physical Review B, 76(9), 094523, 2007.
[32] Ru Y., Yong H., Zhou Y. Fracture analysis of bulk superconductors under electromagnetic force. Engineering Fracture Mechanics, 199:257-273, 2018.
[33] Callister W., Rethwisch D. Material science and engineering - An introduction. John Wiley & Sons, pp. 990, 2007.
[34] van der Laan D.C., Ekin J.W. Large intrinsic effect of axial strain on the critical current of high-temperature superconductors for electric power applications. Applied Physics Letters, 90(5), 052506, 2007.
[35] Duron J., Grilli F., Dutoit B., et al. Modelling the E–J relation of high-Tc superconductors in an arbitrary current range. Physica C: Superconductivity, 401(1-4):231-235, 2004.
[36] Diko P., Krabbes G. Macro-cracking in melt-grown YBaCuO superconductor induced by surface oxygenation. Superconductor Science and Technology, 16(1):90-93, 2002.
[37] Ren Y., Weinstein R., Liu J., et al. Damage caused by magnetic pressure at high trapped field in quasi-permanent magnets composed of melt-textured Y-Ba-Cu-O superconductor. Physica C: Superconductivity, 251(1-2):15-26, 1995.
[38] Hong Z., Campbell A.M., Coombs T.A. Numerical solution of critical state in superconductivity by finite element software. Supercond. Sci. Technol., 19(12):1246-1252, 2006.
Document information
Published on 28/12/23
Accepted on 24/12/23
Submitted on 01/09/23
Volume 39, Issue 4, 2023
DOI: 10.23967/j.rimni.2024.01.003
Licence: CC BY-NC-SA license
Share this document
Keywords
claim authorship
Are you one of the authors of this document?
